Page 83 - Start Up Mathematics_8 (Non CCE)

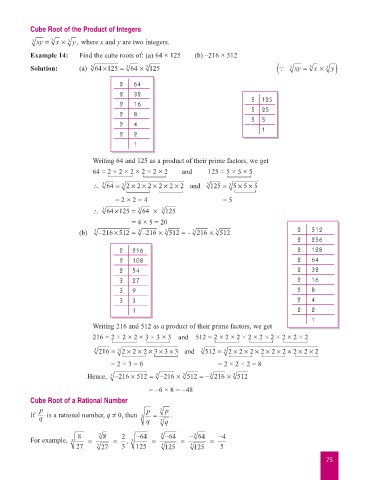
Cube Root of the Product of Integers

3 xy = 3 x ¥ 3 y, where x and y are two integers.

Example 14: Find the cube roots of: (a) 64 × 125 (b) –216 × 512
Solution: (a) 64 125¥ = 3 64 ¥ 3 125 (  3 xy = 3 x ¥ 3 y )
3

2 64
2 32
2 16 5 125
5
25
2 8
2 4 5 5
2 2 1
1

Writing 64 and 125 as a product of their prime factors, we get
64 = 2 × 2 × 2 × 2 × 2 × 2 and 125 = 5 × 5 × 5

\ 64 = 3 2 ¥¥¥¥¥ 2 and 3 125 = 3 555¥ ¥
2
3
2
2
2
= 2 × 2 = 4 = 5
\ 64 125¥ = 3 64 ¥ 3 125
3
= 4 × 5 = 20
¥
3
3
3
3
(b) 3 -216 512 =-216 ¥ 512 = - 216 ¥ 512 2 512
2 256
2 216 2 128
2 108 2 64
2 54 2 32
3 27 2 16
3 9 2 8
3 3 2 4
1 2 2
1
Writing 216 and 512 as a product of their prime factors, we get
216 = 2 × 2 × 2 × 3 × 3 × 3 and 512 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2

3 216 = 3 2 ¥¥ ¥¥¥ 3 512 = 3 2 ¥¥¥¥¥¥¥¥ 2
2
333 and
2
2
2
2
2
2
2
2
= 2 × 3 = 6 = 2 × 2 × 2 = 8
3
3
3
3
Hence, -216 ¥ 512 =-216 ¥ 512 = - 216 ¥ 512
3
= –6 × 8 = –48
Cube Root of a Rational Number
p p 3 p
If q is a rational number, q π 0, then 3 q = 3 q .
8 3 8 2 - 64 3 - 64 - 3 64 - 4
For example, 3 = = , 3 = = =
27 3 27 3 125 3 125 3 125 5
75