Page 338 - Start Up Mathematics_8 (Non CCE)

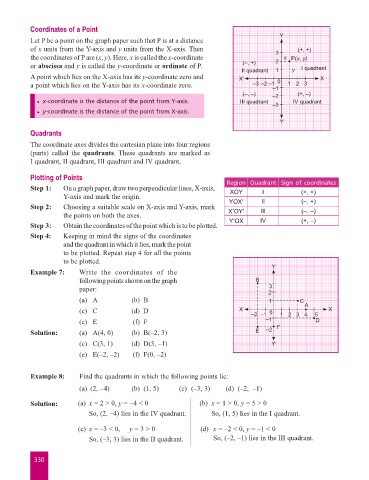
Coordinates of a Point
Y
Let P be a point on the graph paper such that P is at a distance
of x units from the Y-axis and y units from the X-axis. Then 3 (+, +)
the coordinates of P are (x, y). Here, x is called the x-coordinate 2 x P(x, y)
or abscissa and y is called the y-coordinate or ordinate of P. (–, +) I quadrant
II quadrant 1 y
A point which lies on the X-axis has its y-coordinate zero and X’ X
a point which lies on the Y-axis has its x-coordinate zero. –3 –2 –1 –1 0 1 2 3
(–, –) –2 (+, –)
l x-coordinate is the distance of the point from Y-axis. III quadrant IV quadrant
–3
l y-coordinate is the distance of the point from X-axis.

Y’
Quadrants
The coordinate axes divides the cartesian plane into four regions
(parts) called the quadrants. These quadrants are marked as
I quadrant, II quadrant, III quadrant and IV quadrant.

Plotting of Points
Step 1: On a graph paper, draw two perpendicular lines, X-axis, Region Quadrant Sign of coordinates
I
(+, +)
XOY
Y-axis and mark the origin. II (–, +)
YOX¢
Step 2: Choosing a suitable scale on X-axis and Y-axis, mark X¢OY¢ III (–, –)
the points on both the axes.
Step 3: Obtain the coordinates of the point which is to be plotted. Y¢OX IV (+, –)
Step 4: Keeping in mind the signs of the coordinates
and the quadrant in which it lies, mark the point
to be plotted. Repeat step 4 for all the points
to be plotted.
Y
Example 7: Write the coordinates of the
following points shown on the graph B
paper: 3
2
(a) A (b) B 1 C A
(c) C (d) D X’ 0 X
–2 –1 1 2 3 4 5
(e) E (f) F –1 F D
Solution: (a) A(4, 0) (b) B(–2, 3) E –2
(c) C(3, 1) (d) D(5, –1) Y’

(e) E(–2, –2) (f) F(0, –2)

Example 8: Find the quadrants in which the following points lie:

(a) (2, –4) (b) (1, 5) (c) (–3, 3) (d) (–2, –1)

Solution: (a) x = 2 > 0, y = –4 < 0 (b) x = 1 > 0, y = 5 > 0
So, (2, –4) lies in the IV quadrant. So, (1, 5) lies in the I quadrant.

(c) x = –3 < 0, y = 3 > 0 (d) x = –2 < 0, y = –1 < 0
So, (–3, 3) lies in the II quadrant. So, (–2, –1) lies in the III quadrant.

330