Page 105 - Start Up Mathematics_8 (Non CCE)

2
2
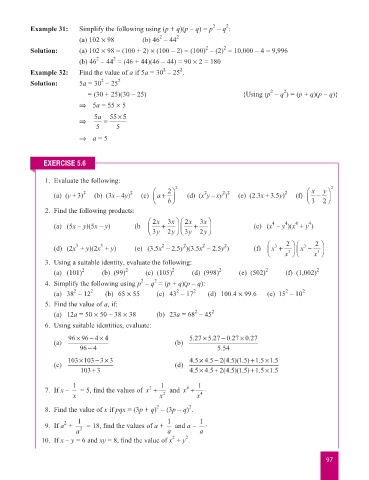
Example 31: Simplify the following using (p + q)(p – q) = p – q :
2
(a) 102 ¥ 98 (b) 46 – 44 2
2
2
Solution: (a) 102 ¥ 98 = (100 + 2) ¥ (100 – 2) = (100) – (2) = 10,000 – 4 = 9,996
2
2
(b) 46 – 44 = (46 + 44)(46 – 44) = 90 ¥ 2 = 180
2
2
Example 32: Find the value of a if 5a = 30 – 25 .
2
Solution: 5a = 30 – 25 2
2
2
= (30 + 25)(30 – 25) {Using (p – q ) = (p + q)(p – q)}
fi 5a = 55 ¥ 5
5a 55 5¥
fi =
5 5
fi a = 5

EXERCISE 5.6

1. Evaluate the following:
ˆ 2
Ê
2 2
2
2
2
2
(a) (y + 3) (b) (3x – 4y) (c) a + b¯ 2 (d) (x y – xy ) (e) (2.3x + 3.5y) (f) Ê Á Ë x - yˆ ˜ 2
˜
Á
2¯
Ë
3
2. Find the following products:
Ê 2x 3x ˆ Ê 2x 3x ˆ 4 4 4 4
(a) (5x – y)(5x – y) (b Á Ë 3y + 2y¯ Ë 3y + 2y¯ ˜ (c) (x – y )(x + y )
˜ Á
2 ˆ Ê
2 ˆ
2
3
2
2
3
2
3
3
(d) (2x + y)(2x + y) (e) (3.5x – 2.5y )(3.5x – 2.5y ) (f) Ê Á Ë x + x ¯ Ë x - x ¯
3 ˜ Á
3 ˜
3. Using a suitable identity, evaluate the following:
2
2
2
2
2
(a) (101) (b) (99) (c) (105) (d) (998) (e) (502) (f) (1,002) 2
2
2
4. Simplify the following using p – q = (p + q)(p – q):
2
2
2
2
2
(a) 38 – 12 (b) 65 ¥ 55 (c) 43 – 17 (d) 100.4 ¥ 99.6 (e) 15 – 10 2
5. Find the value of a, if:
2
(a) 12a = 50 ¥ 50 – 38 ¥ 38 (b) 23a = 68 – 45 2
6. Using suitable identities, evaluate:
96 9644¥ - ¥ 5275 27 0270 27. ¥ . - . ¥ .
(a) (b)
96 4- 554.
103 10333¥ - ¥ 45 45 24 51 5. ¥ . - ( .)(. ) + 1 51 5. ¥ .
(c) (d)
103 3+ 45 45 24 51 5. ¥ . + ( .)(. ) + 1 51 5. ¥ .
1 1 1
7. If x – = 5, find the values of x + and x + .
4
2
x x 2 x 4
2
2
8. Find the value of x if pqx = (3p + q) – (3p – q) .
2
9. If a + 1 = 18, find the values of a + 1 and a – 1 .
a 2 a a
2
2
10. If x – y = 6 and xy = 8, find the value of x + y .
97