Page 100 - Start Up Mathematics_8 (Non CCE)

2
2
2
2
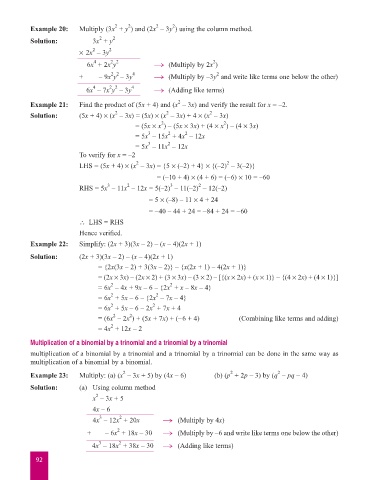
Example 20: Multiply (3x + y ) and (2x – 3y ) using the column method.
2
Solution: 3x + y 2
2
¥ 2x – 3y 2
4
2
2 2
6x + 2x y Æ (Multiply by 2x )
2 2
2
+ – 9x y – 3y 4 Æ (Multiply by –3y and write like terms one below the other)
4
2 2
4
6x – 7x y – 3y Æ (Adding like terms)
2
Example 21: Find the product of (5x + 4) and (x – 3x) and verify the result for x = –2.
2
2
2
Solution: (5x + 4) ¥ (x – 3x) = (5x) ¥ (x – 3x) + 4 ¥ (x – 3x)
2
2
= (5x ¥ x ) – (5x ¥ 3x) + (4 ¥ x ) – (4 ¥ 3x)
2
3
2
= 5x – 15x + 4x – 12x
3
2
= 5x – 11x – 12x
To verify for x = –2
2
2
LHS = (5x + 4) ¥ (x – 3x) = {5 ¥ (–2) + 4} ¥ {(–2) – 3(–2)}
= (–10 + 4) ¥ (4 + 6) = (–6) ¥ 10 = –60
3
3
2
2
RHS = 5x – 11x – 12x = 5(–2) – 11(–2) – 12(–2)
= 5 ¥ (–8) – 11 ¥ 4 + 24
= –40 – 44 + 24 = –84 + 24 = –60
\ LHS = RHS
Hence verified.
Example 22: Simplify: (2x + 3)(3x – 2) – (x – 4)(2x + 1)
Solution: (2x + 3)(3x – 2) – (x – 4)(2x + 1)
= {2x(3x – 2) + 3(3x – 2)} – {x(2x + 1) – 4(2x + 1)}
= (2x ¥ 3x) – (2x ¥ 2) + (3 ¥ 3x) – (3 ¥ 2) – [{(x ¥ 2x) + (x ¥ 1)} – {(4 ¥ 2x) + (4 ¥ 1)}]
2
2
= 6x – 4x + 9x – 6 – {2x + x – 8x – 4}
2
2
= 6x + 5x – 6 – {2x – 7x – 4}
2
2
= 6x + 5x – 6 – 2x + 7x + 4
2
2
= (6x – 2x ) + (5x + 7x) + (–6 + 4) (Combining like terms and adding)
2
= 4x + 12x – 2
Multiplication of a binomial by a trinomial and a trinomial by a trinomial
multiplication of a binomial by a trinomial and a trinomial by a trinomial can be done in the same way as
multiplication of a binomial by a binomial.
2
2
2
Example 23: Multiply: (a) (x – 3x + 5) by (4x – 6) (b) (p + 2p – 3) by (q – pq – 4)
Solution: (a) Using column method
2
x – 3x + 5
4x – 6
3
2
4x – 12x + 20x Æ (Multiply by 4x)
2
+ – 6x + 18x – 30 Æ (Multiply by –6 and write like terms one below the other)
3
2
4x – 18x + 38x – 30 Æ (Adding like terms)
92