Page 106 - Start Up Mathematics_8 (Non CCE)

Ê ˆ 1 2 2 1
11. If x - x¯ ˜ = 36, find the value of x + x 2 .
Á
Ë
1 1
12. If p + = 12, find the value of p – .
p p

13. Find the product:
4
4
2
2
2
2
(a) (2a – 3b)(2a + 3b)(4a + 9b ) (b) (p – q)(p + q)(p + q )(p + q )
1
2
2
(c) (5m – 7n) + (5m + 7n) 2 (d) Ê Á Ë y + 1 ˆ Ê y - 1 ˆ Ê y + y ¯ ˆ ˜
˜ Á
˜ Á
2
y¯ Ë
y¯ Ë
14. Prove that:
2
2
(a) (x + y)(x – y) + (y + z)(y – z) + (z + x)(z – x) = 0 (b) (3x + 4y) – (3x – 4y) = 48xy
2
4
2
15. If x + y = 26 and xy = 3, find the value of : (a) x + y (b) x – y (c) x + y 4
16. If 3x + 4y = 16 and 3x – 4y = 4, find the value of xy.
2
2
[Hint: Use the formula (p + q) – (p – q) = 4pq.]
1
2
2
17. Find the value of 36x + 25y – 60xy when x = 2 , y = .
3 5
MATHS LAB ACTIVITY
2
2
2
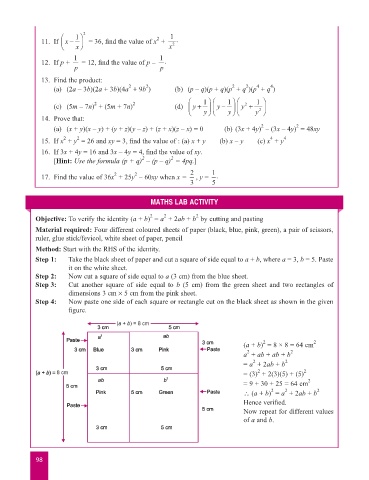
Objective: To verify the identity (a + b) = a + 2ab + b by cutting and pasting
Material required: Four different coloured sheets of paper (black, blue, pink, green), a pair of scissors,
ruler, glue stick/fevicol, white sheet of paper, pencil
Method: Start with the RHS of the identity.
Step 1: Take the black sheet of paper and cut a square of side equal to a + b, where a = 3, b = 5. Paste
it on the white sheet.
Step 2: Now cut a square of side equal to a (3 cm) from the blue sheet.
Step 3: Cut another square of side equal to b (5 cm) from the green sheet and two rectangles of
dimensions 3 cm ¥ 5 cm from the pink sheet.
Step 4: Now paste one side of each square or rectangle cut on the black sheet as shown in the given
figure.

2
(a + b) = 8 × 8 = 64 cm 2
2
a + ab + ab + b 2
2
= a + 2ab + b 2
2
= (3) + 2(3)(5) + (5) 2
= 9 + 30 + 25 = 64 cm 2
2
2
\ (a + b) = a + 2ab + b 2
Hence verified.
Now repeat for different values
of a and b.

98