Page 47 - ICSE Math 5

Multiplication by 1,000 and its multiples

• To multiply a number by 1,000, write the multiplicand followed by three zeros.

• To multiply a number by any multiple of 1,000, write three zeros at the hundreds, tens and
ones places. Then multiply the multiplicand by the remaining digit(s) of the multiplier and
write the product obtained before the three zeros.
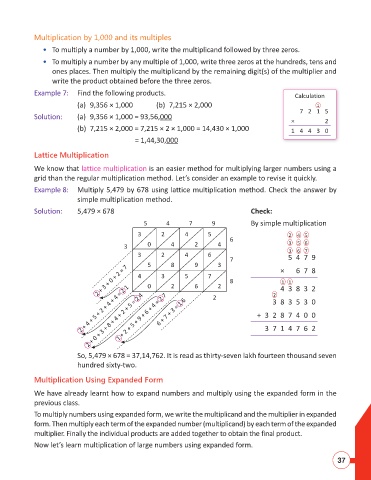
Example 7: Find the following products.
Calculation
(a) 9,356 × 1,000 (b) 7,215 × 2,000 1
7215
Solution: (a) 9,356 × 1,000 = 93,56,000
× 2
(b) 7,215 × 2,000 = 7,215 × 2 × 1,000 = 14,430 × 1,000 1 4430

= 1,44,30,000

Lattice Multiplication

We know that lattice multiplication is an easier method for multiplying larger numbers using a
grid than the regular multiplication method. Let’s consider an example to revise it quickly.
Example 8: Multiply 5,479 by 678 using lattice multiplication method. Check the answer by
simple multiplication method.

Solution: 5,479 × 678 Check:
5 4 7 9 By simple multiplication

3 2 4 5 2 4 5
6
3 0 4 2 4 3 5 6
3 6 7
3 2 4 6 5 4 7 9
7
3
8
5
9
2 + 3 + 0 + 2 = 7 4 0 3 2 5 6 7 2 8 × 6 7 8
1
1
2 + 4 + 5 + 2 + 4 + 4 = 2 1 6 + 7 + 3 = 1 6 2 3 8 3 5 3 0
4 3 8 3 2
2 + 0 + 3 + 8 + 4 + 2 + 5 = 2 4
2
1 + 2 + 5 + 9 + 6 + 4 = 2 7
+ 3 2 8 7 4 0 0
3 7 1 4 7 6 2

So, 5,479 × 678 = 37,14,762. It is read as thirty-seven lakh fourteen thousand seven
hundred sixty-two.
Multiplication Using Expanded Form

We have already learnt how to expand numbers and multiply using the expanded form in the
previous class.
To multiply numbers using expanded form, we write the multiplicand and the multiplier in expanded
form. Then multiply each term of the expanded number (multiplicand) by each term of the expanded
multiplier. Finally the individual products are added together to obtain the final product.
Now let’s learn multiplication of large numbers using expanded form.

37