Page 46 - ICSE Math 5

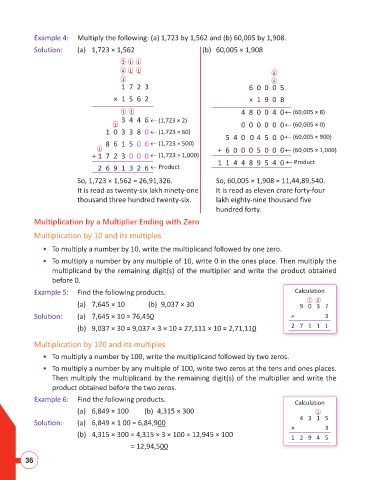
Example 4: Multiply the following: (a) 1,723 by 1,562 and (b) 60,005 by 1,908.
Solution: (a) 1,723 × 1,562 (b) 60,005 × 1,908

3 1 1
4 1 1 4
1 4
1 7 2 3 6 0 0 0 5
× 1 5 6 2 × 1 9 0 8

1 1 4 8 0 0 4 0  (60,005 × 8)
3 4 4 6  (1,723 × 2)
1 0 0 0 0 0 0  (60,005 × 0)
1 0 3 3 8 0 (1,723 × 60)
5 4 0 0 4 5 0 0  (60,005 × 900)
8 6 1 5 0 0 (1,723 × 500)
1 + 6 0 0 0 5 0 0 0 (60,005 × 1,000)
+ 1 7 2 3 0 0 0 (1,723 × 1,000)
1 1 4 4 8 9 5 4 0 Product
2 6 9 1 3 2 6 Product
So, 1,723 × 1,562 = 26,91,326. So, 60,005 × 1,908 = 11,44,89,540.
It is read as twenty-six lakh ninety-one It is read as eleven crore forty-four
thousand three hundred twenty-six. lakh eighty-nine thousand five
hundred forty.
Multiplication by a Multiplier Ending with Zero

Multiplication by 10 and its multiples

• To multiply a number by 10, write the multiplicand followed by one zero.
• To multiply a number by any multiple of 10, write 0 in the ones place. Then multiply the
multiplicand by the remaining digit(s) of the multiplier and write the product obtained
before 0.
Example 5: Find the following products. Calculation
1 2
(a) 7,645 × 10 (b) 9,037 × 30 9037

Solution: (a) 7,645 × 10 = 76,450 × 3
(b) 9,037 × 30 = 9,037 × 3 × 10 = 27,111 × 10 = 2,71,110 2 7111

Multiplication by 100 and its multiples

• To multiply a number by 100, write the multiplicand followed by two zeros.
• To multiply a number by any multiple of 100, write two zeros at the tens and ones places.
Then multiply the multiplicand by the remaining digit(s) of the multiplier and write the
product obtained before the two zeros.

Example 6: Find the following products.
Calculation
(a) 6,849 × 100 (b) 4,315 × 300 1
4315
Solution: (a) 6,849 × 1 00 = 6,84,900
× 3
(b) 4,315 × 300 = 4,315 × 3 × 100 = 12,945 × 100
1 2945
= 12,94,500

36