Page 282 - ICSE Math 8

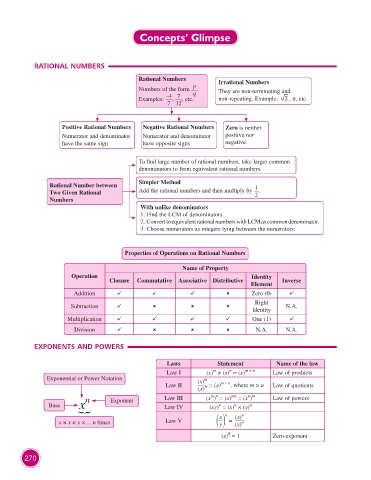
Concepts’ Glimpse

RATIONAL NUMBERS

Rational Numbers Irrational Numbers
Numbers of the form p They are non-terminating and
q
Examples: –4 7 , etc. non-repeating. Example: 2 , p, etc.
,
7 12

Positive Rational Numbers Negative Rational Numbers Zero is neither
Numerator and denominator Numerator and denominator positive nor
have the same sign have opposite signs negative.

To find large number of rational numbers, take larger common
denominators to form equivalent rational numbers.

Rational Number between Simpler Method 1
Two Given Rational Add the rational numbers and then multiply by .
2
Numbers
With unlike denominators
1. Find the LCM of denominators.
2. Convert to equivalent rational numbers with LCM as common denominator.
3. Choose numerators as integers lying between the numerators.

Properties of Operations on Rational Numbers

Name of Property
Operation Identity
Closure Commutative Associative Distributive Inverse
Element
Addition     Zero (0) 
Right
Subtraction     Identity N.A.

Multiplication     One (1) 
Division     N.A. N.A.

EXPONENTS AND POWERS

Laws Statement Name of the law
m
n
Law I (x) × (x) = (x) m + n Law of products
Exponential or Power Notation (x) m
Law II (x) n = (x) m – n , where m > n Law of quotients
n m
mn
m n
x n Exponent Law III (x ) = (x) = (x ) Law of powers
Base Law IV (xy) = (x) × (y) n
n
n
x n (x) n
x × x × x ×… n times Law V y = (y) n
0
(x) = 1 Zero exponent

270