Page 17 - ICSE Math 8


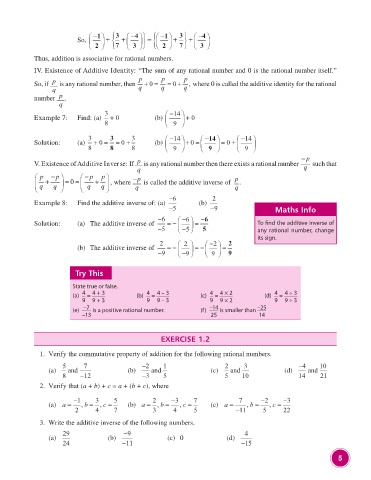
3
So,   −−1  +  3 +  −−4  =     −−1   +  +   −−4  



 2   7  3    2  7   3 
Thus, addition is associative for rational numbers.
IV. Existence of Additive Identity: “The sum of any rational number and 0 is the rational number itself.”
0
0
So, if p is any rational number, then p += p =+ p , where 0 is called the additive identity for the rational
q q q q
number p .
q
3  −14 
Example 7: Find: (a) + 0 (b)   + 0
8  9 

3 3 3  −14   −−14   −14 
Solution: (a) += =+ (b)   +=   = 0+  
0
0
0
8 8 8  9   9   9 
V. Existence of Additive Inverse: If p is any rational number then there exists a rational number − p such that
q q
 p − p   − p p  –p p
 q + q  ==0  q + q  , where is called the additive inverse of .
    q q
−6 2
Example 8: Find the additive inverse of: (a) (b)
−5 − 9 Maths Info
−6  6 −6
− 
Solution: (a) The additive inverse of =−   = To find the additive inverse of
− 
−5  5 5 any rational number, change
its sign.
2  2   − 2  2
(b) The additive inverse of =−   =−   =
− 9  − 9   9  9

Try This

State true or false.
4
(a) = 4 + 3 (b) 4 = 4 – 3 (c) 4 = 4 × 2 (d) 4 = 4 ÷ 3
9 9 + 3 9 9 – 3 9 9 × 2 9 9 ÷ 3
(e) –7 is a positive rational number. (f) –14 is smaller than –25
–13 25 14

EXERCISE 1.2

1. Verify the commutative property of addition for the following rational numbers.

5 7 −2 1 2 3 −4 10
(a) and (b) and (c) and (d) and
8 − 12 −3 5 5 10 14 21
2. Verify that (a + b) + c = a + (b + c), where

−1 3 5 2 −3 7 7 −2 −3
(a) a = , b = , c = (b) a = , b = , c = (c) a = , b = , c =
2 4 7 3 4 5 −11 5 22
3. Write the additive inverse of the following numbers.

29 −9 4
(a) (b) (c) 0 (d)
24 −11 − 15

5