Page 133 - ICSE Math 5

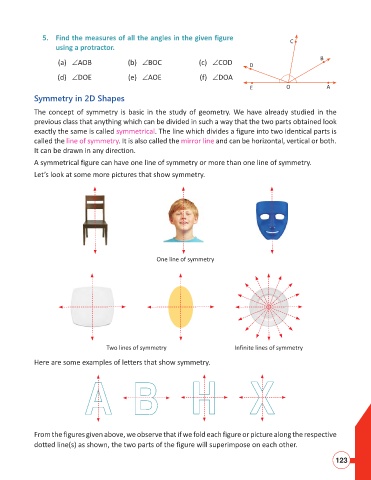
5. Find the measures of all the angles in the given figure
C
using a protractor.
B
(a) AOB (b) BOC (c) COD D

(d) DOE (e) AOE (f) DOA
E O A
Symmetry in 2D Shapes

The concept of symmetry is basic in the study of geometry. We have already studied in the
previous class that anything which can be divided in such a way that the two parts obtained look
exactly the same is called symmetrical. The line which divides a figure into two identical parts is
called the line of symmetry. It is also called the mirror line and can be horizontal, vertical or both.
It can be drawn in any direction.

A symmetrical figure can have one line of symmetry or more than one line of symmetry.
Let’s look at some more pictures that show symmetry.

One line of symmetry

Two lines of symmetry Infinite lines of symmetry

Here are some examples of letters that show symmetry.

From the figures given above, we observe that if we fold each figure or picture along the respective
dotted line(s) as shown, the two parts of the figure will superimpose on each other.

123