Page 55 - Start Up Mathematics_8 (Non CCE)

(iv) When a and b both are even natural numbers.
For example, a = 2, b = 4
2
2
2
2
2
a = (2) = 4, b = (4) = 16, 2b = 2 × 16 = 32
2
Since, 4 π 32 \ a π 2b 2
Pythagorean Triplet
2
2
2
If three natural numbers a, b, c satisfy the condition a + b = c , then the three natural numbers are said to
2
2
2
2
2
2
form a Pythagorean triplet. For example, 3 + 4 = 5 , 8 + 15 = 17 and so on.
2
2
XII. For any natural number a (a > 1), there exists a Pythagorean triplet (2a, a – 1, a + 1).
For example, let the natural number a be 7 (7 > 1).
2
2
Now, 2a = 2 × 7 = 14 a – 1 = 49 – 1 = 48 a + 1 = 49 + 1 = 50
So, Pythagorean triplet is 14, 48, 50.
If a and b are relatively prime (or co-prime) natural numbers (a > b) and one of them is even and the other
2
2
2
2
is odd then the Pythagorean triplet is formed by (2ab, a – b , a + b ).
For example, if a = 8, b = 5, (8 > 5)
2
2
2
2
2
2
2
2
Now 2ab = 2 × 8 × 5 = 80 a – b = 8 – 5 = 64 – 25 = 39 a + b = 8 + 5 = 64 + 25 = 89
Hence, the Pythagorean triplet is 80, 39, 89.
Patterns of Perfect Squares
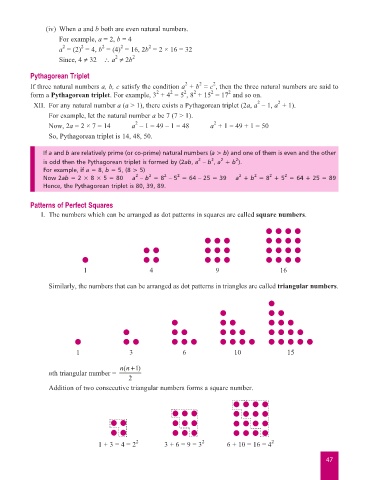
I. The numbers which can be arranged as dot patterns in squares are called square numbers.
1 4 9 16
Similarly, the numbers that can be arranged as dot patterns in triangles are called triangular numbers.

1 3 6 10 15

( nn + 1)
nth triangular number =
2
Addition of two consecutive triangular numbers forms a square number.

1 + 3 = 4 = 2 2 3 + 6 = 9 = 3 2 6 + 10 = 16 = 4 2

47