Page 53 - Start Up Mathematics_8 (Non CCE)

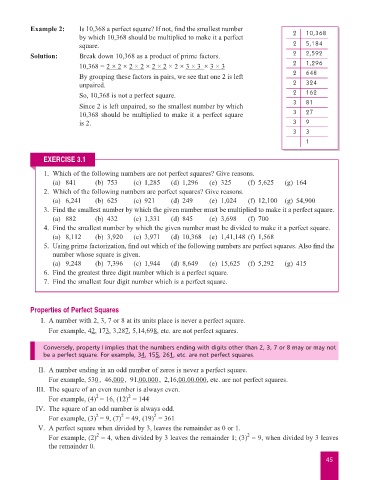
Example 2: Is 10,368 a perfect square? If not, find the smallest number 2 10,368
by which 10,368 should be multiplied to make it a perfect
square. 2 5,184
Solution: Break down 10,368 as a product of prime factors. 2 2,592
10,368 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 3 × 3 × 3 × 3 2 1,296
By grouping these factors in pairs, we see that one 2 is left 2 648
unpaired. 2 324
So, 10,368 is not a perfect square. 2 162
Since 2 is left unpaired, so the smallest number by which 3 81
10,368 should be multiplied to make it a perfect square 3 27
is 2. 3 9
3 3
1

EXERCISE 3.1

1. Which of the following numbers are not perfect squares? Give reasons.
(a) 841 (b) 753 (c) 1,285 (d) 1,296 (e) 325 (f) 5,625 (g) 164
2. Which of the following numbers are perfect squares? Give reasons.
(a) 6,241 (b) 625 (c) 921 (d) 249 (e) 1,024 (f) 12,100 (g) 54,900
3. Find the smallest number by which the given number must be multiplied to make it a perfect square.
(a) 882 (b) 432 (c) 1,331 (d) 845 (e) 3,698 (f) 700
4. Find the smallest number by which the given number must be divided to make it a perfect square.
(a) 8,112 (b) 3,920 (c) 3,971 (d) 10,368 (e) 1,41,148 (f) 1,568
5. Using prime factorization, find out which of the following numbers are perfect squares. Also find the
number whose square is given.
(a) 9,248 (b) 7,396 (c) 1,944 (d) 8,649 (e) 15,625 (f) 5,292 (g) 415
6. Find the greatest three digit number which is a perfect square.
7. Find the smallest four digit number which is a perfect square.

Properties of Perfect Squares
I. A number with 2, 3, 7 or 8 at its units place is never a perfect square.
For example, 42, 173, 3,287, 5,14,698, etc. are not perfect squares.

Conversely, property I implies that the numbers ending with digits other than 2, 3, 7 or 8 may or may not
be a perfect square. For example, 34, 155, 261, etc. are not perfect squares.

II. A number ending in an odd number of zeros is never a perfect square.
For example, 530 , 46,000 , 91,00,000 , 2,16,00,00,000, etc. are not perfect squares.
III. The square of an even number is always even.
2
2
For example, (4) = 16, (12) = 144
IV. The square of an odd number is always odd.
2
2
2
For example, (3) = 9, (7) = 49, (19) = 361
V. A perfect square when divided by 3, leaves the remainder as 0 or 1.
2
2
For example, (2) = 4, when divided by 3 leaves the remainder 1; (3) = 9, when divided by 3 leaves
the remainder 0.
45