Page 39 - Start Up Mathematics_8 (Non CCE)

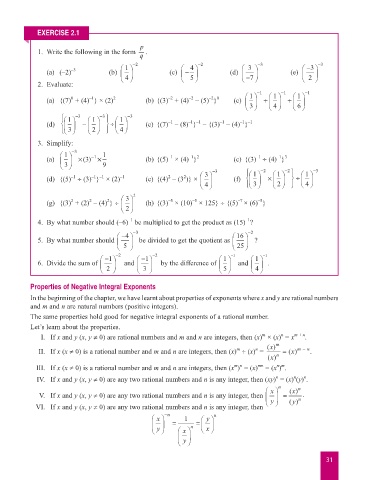
EXERCISE 2.1

p
1. Write the following in the form .
q
− 
−
(a) (–2) –3 (b)  1  − 2 (c)  4  −2 (d)  − 3  − 3 (e)  3   −3



 


 2
 5
7 



4 
2. Evaluate:
1 
1 
2
–1
–2
–2
–2 0
0
(a) {(7) + (4) } × (2) (b) {(3) + (4) – (5) } (c)  1  − 1 +    − 1 +    − 1
 
 3   4   6 
3
   1  − 3  1     − 3

−
1
–1
–1 –1
–1
–1 –1
(d)    −    ÷   (e) {(7) – (8) } – {(3) – (4) }
   3   2     
4
3. Simplify:
 1  − 3 − 1 1
–1
–1
–1 3
–1 2
(a)   × 3 () × (b) {(5) × (4) } (c) {(3) ÷ (4) }
 3  9 − 3 − 2 − 2 − 3

1    
1 
2
2
–1 –1
–1
 ÷  
(d) {(5) ÷ (3) } × (2) –1 (e) {(4) – (3 )} ×  3  (f)      ×     1
 
4
 4     3   2     
2
–7
–5
2
–5
–5
2
(g) {(3) + (2) – (4) } ÷  3  2 (h) {(3) × (10) × 125} ÷ {(5) × (6) }
 
 2 
–1
–1
4. By what number should (–6) be multiplied to get the product as (15) ?
 4 −3  16  − 2
− 
5. By what number should   be divided to get the quotient as   ?
 5   25 
 −  1 −2  −  1 −2  1  − 1  1  − 1
6. Divide the sum of   and   by the difference of   and   .
 2   3   5   4 
Properties of Negative Integral Exponents
In the beginning of the chapter, we have learnt about properties of exponents where x and y are rational numbers
and m and n are natural numbers (positive integers).
The same properties hold good for negative integral exponents of a rational number.
Let’s learn about the properties.
m
n
I. If x and y (x, y ≠ 0) are rational numbers and m and n are integers, then (x) × (x) = x m + n .
()x m
m
n
II. If x (x ≠ 0) is a rational number and m and n are integers, then (x) ÷ (x) = = ()x m − n .
()x n
n m
mn
m n
III. If x (x ≠ 0) is a rational number and m and n are integers, then (x ) = (x) = (x ) .
n
n
n
IV. If x and y (x, y ≠ 0) are any two rational numbers and n is any integer, then (xy) = (x) (y) .
 x  n x () n
V. If x and y (x, y ≠ 0) are any two rational numbers and n is any integer, then   = n .
y
VI. If x and y (x, y ≠ 0) are any two rational numbers and n is any integer, then   y ()
 x  − n 1  y  n
  = n =  
y
   x   x 
 
y
 
31