Page 204 - Start Up Mathematics_8 (Non CCE)

Inverse Problems on Compound Interest

Computing the principal when the amount, C.I., R and n are given
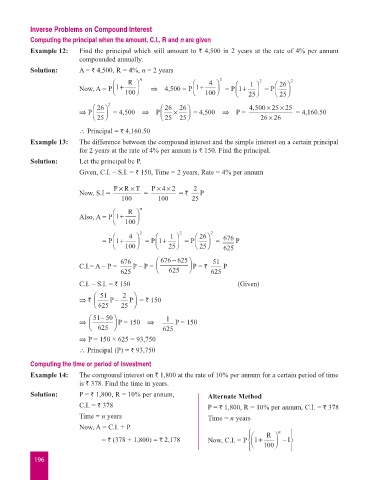
Example 12: Find the principal which will amount to ` 4,500 in 2 years at the rate of 4% per annum
compounded annually.

Solution: A = ` 4,500, R = 4%, n = 2 years
Ê R ˆ n Ê 4 ˆ 2 Ê 1 ˆ 2 Ê 26ˆ 2
Now, A = PÁ Ë 1+ 100¯ ˜ fi 4,500 = PÁ Ë 1+ 100¯ ˜ = P 1+ 25¯ ˜ = P Á 25¯ ˜
Á
Ë
Ë
Ê 26ˆ 2 Ê 26 26ˆ 4 500 25 25, ¥ ¥
fi P Á Ë 25¯ = 4,500 fi P Á Ë 25 ¥ 25¯ = 4,500 fi P = 26 26¥ = 4,160.50
˜
˜
\ Principal = ` 4,160.50
Example 13: The difference between the compound interest and the simple interest on a certain principal
for 2 years at the rate of 4% per annum is ` 150. Find the principal.
Solution: Let the principal be P.

Given, C.I. – S.I. = ` 150, Time = 2 years, Rate = 4% per annum

PR T¥ ¥ P ¥¥42 2
Now, S.I = = = ` P
100 100 25

Ê R ˆ n
Also, A = P 1+ 100¯ ˜
Á
Ë
Ê 4 ˆ 2 Ê 1 ˆ 2 Ê 26ˆ 2 676
= P 1+ 100¯ ˜ = P 1+ 25¯ = P Á Ë 25¯ ˜ = 625 P
Á
Á
˜
Ë
Ë
676 Ê 676 625- ˆ 51
C.I.= A – P = P – P = Á ˜ P = ` P
625 Ë 625 ¯ 625
C.I. – S.I. = ` 150 (Given)
Ê 51 2 ˆ
fi ` Á 625 P - 25 ¯
P = ` 150
˜
Ë
Ê 51 50- ˆ 1
fi Á Ë 625 ¯ ˜ P = 150 fi 625 P = 150

fi P = 150 × 625 = 93,750
\ Principal (P) = ` 93,750

Computing the time or period of investment
Example 14: The compound interest on ` 1,800 at the rate of 10% per annum for a certain period of time
is ` 378. Find the time in years.
Solution: P = ` 1,800, R = 10% per annum, Alternate Method

C.I. = ` 378 P = ` 1,800, R = 10% per annum, C.I. = ` 378
Time = n years Time = n years

Now, A = C.I. + P
Ï Ê Ô R ˆ n ¸
Ô
= ` (378 + 1,800) = ` 2,178 Now, C.I. = P Ì Á 1+ ˜ - 1 ˝

Ó Ô Ë 100¯ ˛ Ô
196