Page 201 - Start Up Mathematics_8 (Non CCE)

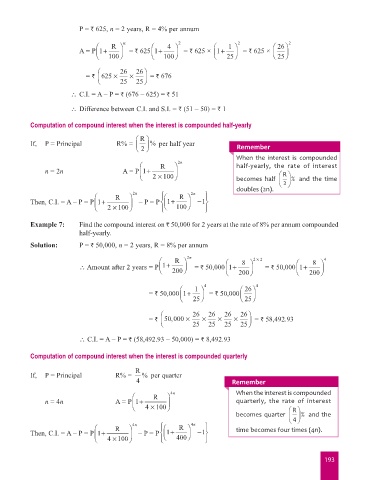
P = ` 625, n = 2 years, R = 4% per annum

Ê R ˆ n Ê 4 ˆ 2 Ê 1 ˆ 2 Ê 26ˆ 2
A = P 1+ 100¯ ˜ = ` 625 1+ 100¯ ˜ = ` 625 × 1+ 25¯ = ` 625 × Á 25¯ ˜
˜
Á
Á
Á
Ë
Ë
Ë
Ë
Ê 26 26ˆ
= ` 625 ¥ 25 ¥ 25¯ = ` 676
˜
Á
Ë
\ C.I. = A – P = ` (676 – 625) = ` 51
\ Difference between C.I. and S.I. = ` (51 – 50) = ` 1
Computation of compound interest when the interest is compounded half-yearly
Ê R ˆ
If, P = Principal R% = Á Ë 2 ¯ ˜ % per half year Remember

When the interest is compounded
Ê R ˆ 2n half-yearly, the rate of interest
n = 2n A = P 1+ 2 100¥ ˜ becomes half  R  % and the time
Á
Ë
¯
2
 
doubles (2n).  
Ê R ˆ 2n Ï Ê Ô R ˆ 2n ¸
Ô
Then, C.I. = A – P = P 1+ 2 100¥ ˜ – P = PÌ Á Ë 1+ 100¯ ˜ - 1 ˝
Á
Ë
¯
˛ Ô
Ó Ô
Example 7: Find the compound interest on ` 50,000 for 2 years at the rate of 8% per annum compounded
half-yearly.
Solution: P = ` 50,000, n = 2 years, R = 8% per annum
Ê R ˆ 2n Ê 8 ˆ 2 ¥ 2 Ê 8 ˆ 4
˜ = ` 50,000 1+
\ Amount after 2 years = PÁ Ë 1+ 200¯ Á Ë 200¯ ˜ = ` 50,000 1+ 200¯
Á
˜
Ë
Ê 1 ˆ 4 Ê 26ˆ 4
= ` 50,000 1+ 25¯ = ` 50,000 Á Ë 25¯ ˜
˜
Á
Ë
Ê 26 26 26 26ˆ
= ` 50 000, ¥ 25 ¥ 25 ¥ 25 ¥ 25¯ ˜ = ` 58,492.93
Á
Ë
\ C.I. = A – P = ` (58,492.93 – 50,000) = ` 8,492.93

Computation of compound interest when the interest is compounded quarterly

R
If, P = Principal R% = % per quarter
4 Remember

Ê R ˆ 4n When the interest is compounded
n = 4n A = P 1+ 4 100¥ ˜ quarterly, the rate of interest
Á
Ë
¯
R 
becomes quarter    % and the
4
 
Ô
Ê R ˆ 4n Ï Ê Ô R ˆ 4n ¸ time becomes four times (4n).
Then, C.I. = A – P = P 1+ 4 100¥ ˜ ¯ – P = P Á Ì Ë 1+ 400¯ ˜ - 1 ˝
Á
Ë
Ó Ô
˛ Ô
193