Page 203 - Start Up Mathematics_8 (Non CCE)

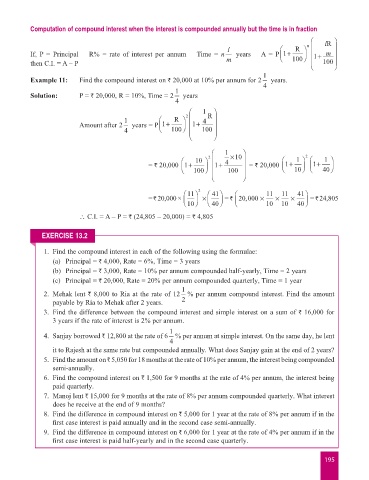
Computation of compound interest when the interest is compounded annually but the time is in fraction

Ê R l ˆ
n
l Ê R ˆ Á ˜
If, P = Principal R% = rate of interest per annum Time = n years A = P 1+ 100¯ 1+ m ˜
Á
˜ Á
Ë
then C.I. = A – P m Á Ë 100 ˜
¯
1
Example 11: Find the compound interest on ` 20,000 at 10% per annum for 2 years.
4
1
Solution: P = ` 20,000, R = 10%, Time = 2 years
4
Ê 1 R ˆ
2
1 Ê R ˆ Á 4 ˜
Amount after 2 years = P 1+ ˜ Á 1+ ˜
Á
4 Ë 100¯ Á Ë 100 ˜
¯
Ê 1 ¥ 10 ˆ 2
2
Ê
1 ˆ
1 ˆ Ê
10 ˆ Á
Ê
= ` 20,000 1+ 100¯ ˜ Á 1+ 4 ˜ ˜ = ` 20,000 1+ 10¯ Ë 1+ 40¯
Á
˜
˜ Á
Á
Ë
Ë
Á Ë 100 ˜ ¯
Ê 11ˆ 2 Ê 41ˆ Ê 11 11 41ˆ
= ` 20,000 × Á Ë 10¯ ˜ ¥ Á Ë 40¯ ˜ = ` 20 000, ¥ 10 ¥ 10 ¥ 40¯ ˜ = ` 24,805
Á
Ë
\ C.I. = A – P = ` (24,805 – 20,000) = ` 4,805
EXERCISE 13.2
1. Find the compound interest in each of the following using the formulae:
(a) Principal = ` 4,000, Rate = 6%, Time = 3 years
(b) Principal = ` 3,000, Rate = 10% per annum compounded half-yearly, Time = 2 years
(c) Principal = ` 20,000, Rate = 20% per annum compounded quarterly, Time = 1 year
1
2. Mehak lent ` 8,000 to Ria at the rate of 12 % per annum compound interest. Find the amount
payable by Ria to Mehak after 2 years. 2
3. Find the difference between the compound interest and simple interest on a sum of ` 16,000 for
3 years if the rate of interest is 2% per annum.
1
4. Sanjay borrowed ` 12,800 at the rate of 6 % per annum at simple interest. On the same day, he lent
4
it to Rajesh at the same rate but compounded annually. What does Sanjay gain at the end of 2 years?
5. Find the amount on ` 5,050 for 18 months at the rate of 10% per annum, the interest being compounded
semi-annually.
6. Find the compound interest on ` 1,500 for 9 months at the rate of 4% per annum, the interest being
paid quarterly.
7. Manoj lent ` 15,000 for 9 months at the rate of 8% per annum compounded quarterly. What interest
does he receive at the end of 9 months?
8. Find the difference in compound interest on ` 5,000 for 1 year at the rate of 8% per annum if in the
first case interest is paid annually and in the second case semi-annually.
9. Find the difference in compound interest on ` 6,000 for 1 year at the rate of 4% per annum if in the
first case interest is paid half-yearly and in the second case quarterly.

195