Page 55 - Viva

Example 1: Construct the table of 7 by skip counting.

Solution: 7 14 21 28 35 42 49 56 63 70

+ 7 + 7 + 7 + 7 + 7 + 7 + 7 + 7 + 7

Through Grid Patterns
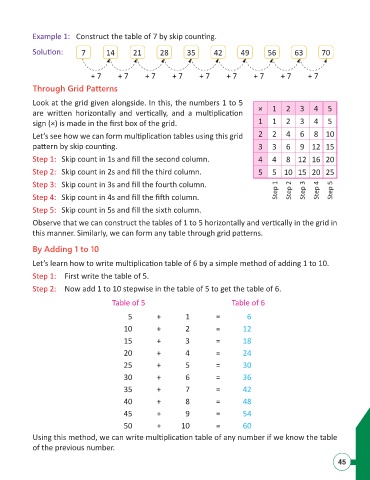
Look at the grid given alongside. In this, the numbers 1 to 5
are written horizontally and vertically, and a multiplication × 1 2 3 4 5
sign (×) is made in the first box of the grid. 1 1 2 3 4 5

Let’s see how we can form multiplication tables using this grid 2 2 4 6 8 10
pattern by skip counting. 3 3 6 9 12 15

Step 1: Skip count in 1s and fill the second column. 4 4 8 12 16 20
Step 2: Skip count in 2s and fill the third column. 5 5 10 15 20 25

Step 3: Skip count in 3s and fill the fourth column.

Step 4: Skip count in 4s and fill the fifth column. Step 1 Step 2 Step 3 Step 4 Step 5
Step 5: Skip count in 5s and fill the sixth column.

Observe that we can construct the tables of 1 to 5 horizontally and vertically in the grid in
this manner. Similarly, we can form any table through grid patterns.

By Adding 1 to 10
Let’s learn how to write multiplication table of 6 by a simple method of adding 1 to 10.

Step 1: First write the table of 5.
Step 2: Now add 1 to 10 stepwise in the table of 5 to get the table of 6.

Table of 5 Table of 6

5 + 1 = 6

10 + 2 = 12
15 + 3 = 18

20 + 4 = 24
25 + 5 = 30

30 + 6 = 36
35 + 7 = 42

40 + 8 = 48
45 + 9 = 54

50 + 10 = 60
Using this method, we can write multiplication table of any number if we know the table
of the previous number.

45