Page 71 - Start Up Mathematics_7

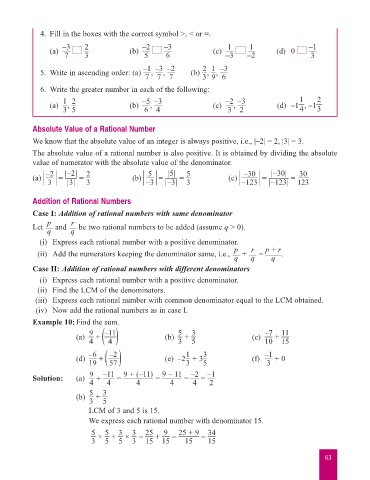
4. Fill in the boxes with the correct symbol >, < or =.
–3 2 –2 –3 1 1 –1
(a) (b) (c) (d) 0
7 3 5 6 –3 –2 3

–1 –3 –2 2 1 –3
5. Write in ascending order: (a) , , (b) , ,
7 7 7 3 9 6
6. Write the greater number in each of the following:

1 2 –5 –3 –2 –3 1 2
(a) , (b) , (c) , (d) –1 , –1
3 5 6 4 3 2 4 3

Absolute Value of a Rational Number
We know that the absolute value of an integer is always positive, i.e., |–2| = 2, |3| = 3.
The absolute value of a rational number is also positive. It is obtained by dividing the absolute
value of numerator with the absolute value of the denominator.

–2 |–2| 2 5 |5| 5 –30 |–30| 30
(a) = = (b) = = (c) = =
3 |3| 3 –3 |–3| 3 –123 |–123| 123

Addition of Rational Numbers

Case I: Addition of rational numbers with same denominator
p r
Let and be two rational numbers to be added (assume q > 0).
q q
(i) Express each rational number with a positive denominator.
(ii) Add the numerators keeping the denominator same, i.e., p + r = p + r .
q q q
Case II: Addition of rational numbers with different denominators

(i) Express each rational number with a positive denominator.
(ii) Find the LCM of the denominators.
(iii) Express each rational number with common denominator equal to the LCM obtained.
(iv) Now add the rational numbers as in case I.
Example 10: Find the sum.

9 –11 5 3 –7 11
(a) + (b) + (c) +
4 4 3 5 10 15
–6 –2 1 3 –1
(d) + (e) –2 + 3 (f) + 0
19 57 3 5 3
9 –11 9 + (–11) 9 – 11 –2 –1
Solution: (a) + = = = =
4 4 4 4 4 2
5 3
(b) +
3 5
LCM of 3 and 5 is 15.
We express each rational number with denominator 15.
3
5 × + × = 25 + 9 = 25 + 9 = 34
5
3
3 5 5 3 15 15 15 15
63