Page 67 - Start Up Mathematics_7

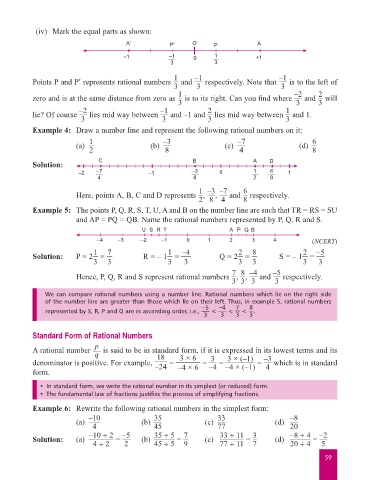
(iv) Mark the equal parts as shown:

A′ P′ O P A

–1 –1 0 1 +1
3 3

1 –1 –1
Points P and P′ represents rational numbers and respectively. Note that is to the left of
3 3 3
1
2
zero and is at the same distance from zero as is to its right. Can you find where –2 and will
3
3
3
–2 –1 2 1
lie? Of course lies mid way between and –1 and lies mid way between and 1.
3 3 3 3
Example 4: Draw a number line and represent the following rational numbers on it:
(a) 1 (b) –3 (c) –7 (d) 6
2 8 4 8

Solution: C B A D
–2 –7 –1 –3 0 1 6 1
4 8 2 8
1 –3 –7 6
Here, points A, B, C and D represents , , and respectively.
2 8 4 8
Example 5: The points P, Q, R, S, T, U, A and B on the number line are such that TR = RS = SU
and AP = PQ = QB. Name the rational numbers represented by P, Q, R and S.

U S R T A P Q B
–4 –3 –2 –1 0 1 2 3 4 (NCERT)
1 7 1 –4 2 8 2 –5
Solution: P = 2 = R = – 1 = Q = 2 = S = – 1 =
3 3 3 3 3 3 3 3
7 8 –4 –5
Hence, P, Q, R and S represent rational numbers , , and respectively.
3 3 3 3

We can compare rational numbers using a number line. Rational numbers which lie on the right side
of the number line are greater than those which lie on their left. Thus, in example 5, rational numbers
7
8
represented by S, R, P and Q are in ascending order, i.e., –5 < –4 < < .
3
3
3 3
Standard Form of Rational Numbers
p
A rational number is said to be in standard form, if it is expressed in its lowest terms and its
q 18 3 × 6
3 × (–1)
3
denominator is positive. For example, –24 = –4 × 6 = –4 = –4 × (–1) = –3 which is in standard
4
form.
• In standard form, we write the rational number in its simplest (or reduced) form.
• The fundamental law of fractions justifies the process of simplifying fractions.

Example 6: Rewrite the following rational numbers in the simplest form:

(a) –10 (b) 35 (c) 33 (d) –8
4 45 77 20
–10 ÷ 2 –5 35 ÷ 5 7 33 ÷ 11 3 –8 ÷ 4 –2
Solution: (a) = (b) = (c) = (d) =
4 ÷ 2 2 45 ÷ 5 9 77 ÷ 11 7 20 ÷ 4 5

59