Page 69 - Start Up Mathematics_7

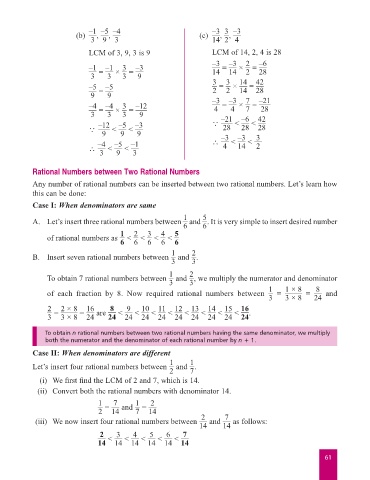
–1 –5 –4 –3 3 –3
(b) , , (c) , ,
3 9 3 14 2 4

LCM of 3, 9, 3 is 9 LCM of 14, 2, 4 is 28
–3 –3 2 –6
3
–1 = –1 × = –3 14 = 14 × = 28
2
3 3 3 9
3
–5 = –5 3 = × 14 = 42
28
2
2
14
9 9 –3 –3 7 –21
–4 = –4 × = –12 4 = 4 × = 28
3
7
3 3 3 9 –21 –6 42
–12 –5 –3  < <
 < < 28 28 28
9 9 9 –3 –3 3
–4 –5 –1 ∴ 4 < 14 < 2
∴ < <
3 9 3
Rational Numbers between Two Rational Numbers

Any number of rational numbers can be inserted between two rational numbers. Let’s learn how
this can be done:
Case I: When denominators are same

1 5
A. Let’s insert three rational numbers between and . It is very simple to insert desired number
6
6
1 2 3 4 5
of rational numbers as < < < < 6
6
6
6
6
1 2
B. Insert seven rational numbers between and .
3 3
1 2
To obtain 7 rational numbers between and , we multiply the numerator and denominator
3 3
1
of each fraction by 8. Now required rational numbers between = 1 × 8 = 8 and
3 3 × 8 24
2 = 2 × 8 = 16 are 8 < 9 < 10 < 11 < 12 < 13 < 14 < 15 < 16 .
3 3 × 8 24 24 24 24 24 24 24 24 24 24

To obtain n rational numbers between two rational numbers having the same denominator, we multiply
both the numerator and the denominator of each rational number by n + 1.

Case II: When denominators are different
1 1
Let’s insert four rational numbers between and .
2 7
(i) We first find the LCM of 2 and 7, which is 14.

(ii) Convert both the rational numbers with denominator 14.
1 7 1 2
= and =
2 14 7 14
(iii) We now insert four rational numbers between 2 and 7 as follows:
14 14
2 3 4 5 6 7
< < < < <
14 14 14 14 14 14

61