Page 74 - Start Up Mathematics_7

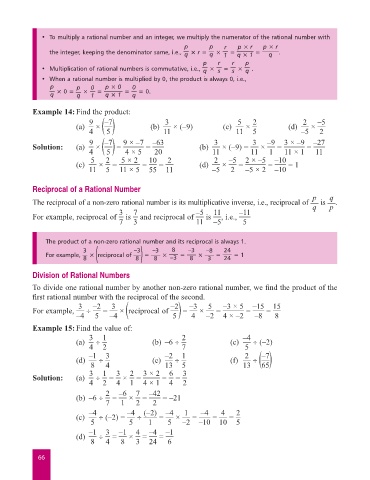
• To multiply a rational number and an integer, we multiply the numerator of the rational number with
p p r p × r p × r
the integer, keeping the denominator same, i.e., × r = × = q × 1 = q .
q
q
1
p r r p
• Multiplication of rational numbers is commutative, i.e., × = × .
s
q
q
s
• When a rational number is multiplied by 0, the product is always 0, i.e.,
p p 0 p × 0 0
q × 0 = × = q × 1 = = 0.
q
q
1
Example 14: Find the product:
9 –7 3 5 2 2 –5
(a) × (b) × (–9) (c) × (d) ×
4 5 11 11 5 –5 2
9 –7 9 × –7 –63 3 3 –9 3 × –9 –27
Solution: (a) × = = (b) × (–9) = × = =
4 5 4 × 5 20 11 11 1 11 × 1 11
5 2 5 × 2 10 2 2 –5 2 × –5 –10
(c) × = = = (d) × = = = 1
11 5 11 × 5 55 11 –5 2 –5 × 2 –10

Reciprocal of a Rational Number
p q
The reciprocal of a non-zero rational number is its multiplicative inverse, i.e., reciprocal of is p .
q
3 7 –5 11 –11
For example, reciprocal of is and reciprocal of is , i.e.,
7 3 11 –5 5

The product of a non-zero rational number and its reciprocal is always 1.
3 –3 –3 8 –3 –8 24
For example, × reciprocal of = × = × = = 1
8 8 8 –3 8 3 24

Division of Rational Numbers
To divide one rational number by another non-zero rational number, we find the product of the
first rational number with the reciprocal of the second.
3 –2 3 –2 –3 5 –3 × 5 –15 15
For example, ÷ = × reciprocal of = × = = =
–4 5 –4 5 4 –2 4 × –2 –8 8

Example 15: Find the value of:
3 1 2 –4
(a) ÷ (b) –6 ÷ (c) ÷ (–2)
4 2 7 5
–1 3 –2 1 2 –7
(d) ÷ (e) ÷ (f) ÷
8 4 13 5 13 65
3 1 3 2 3 × 2 6 3
Solution: (a) ÷ = × = = =
4 2 4 1 4 × 1 4 2
2 –6 7 –42
(b) –6 ÷ = × = = –21
7 1 2 2
–4 –4 (–2) –4 1 –4 4 2
(c) ÷ (–2) = ÷ = × = = =
5 5 1 5 –2 –10 10 5
–1 3 –1 4 –4 –1
(d) ÷ = × = =
8 4 8 3 24 6

66