Page 228 - Start Up Mathematics_7

180° 180°
O O
O

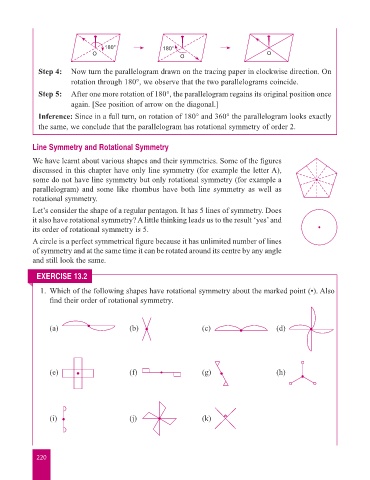
Step 4: Now turn the parallelogram drawn on the tracing paper in clockwise direction. On
rotation through 180°, we observe that the two parallelograms coincide.
Step 5: After one more rotation of 180°, the parallelogram regains its original position once
again. [See position of arrow on the diagonal.]

Inference: Since in a full turn, on rotation of 180° and 360° the parallelogram looks exactly
the same, we conclude that the parallelogram has rotational symmetry of order 2.

Line Symmetry and Rotational Symmetry

We have learnt about various shapes and their symmetries. Some of the figures
discussed in this chapter have only line symmetry (for example the letter A),
some do not have line symmetry but only rotational symmetry (for example a
parallelogram) and some like rhombus have both line symmetry as well as
rotational symmetry.
Let’s consider the shape of a regular pentagon. It has 5 lines of symmetry. Does
it also have rotational symmetry? A little thinking leads us to the result ‘yes’ and
its order of rotational symmetry is 5.

A circle is a perfect symmetrical figure because it has unlimited number of lines
of symmetry and at the same time it can be rotated around its centre by any angle
and still look the same.

EXERCISE 13.2

1. Which of the following shapes have rotational symmetry about the marked point (•). Also
find their order of rotational symmetry.

(a) (b) (c) (d)

(e) (f) (g) (h)

(i) (j) (k)

220