Page 225 - Start Up Mathematics_7

Rotation

Rotation is the circular movement of an object about a point. In our day-to-day life, we come
across objects which rotate around a point, such as a bicycle wheel, blades of a ceiling fan, blades
of a windmill, etc. The fixed point about which the object rotates is called the centre of rotation.
There are two types of rotation.
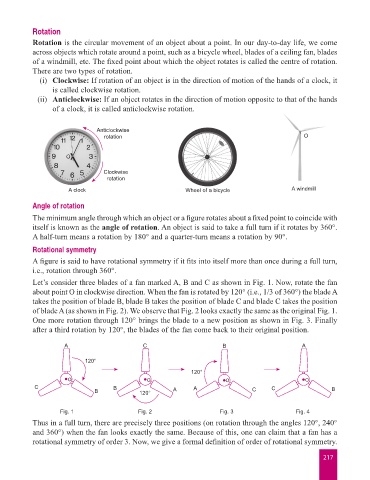
(i) Clockwise: If rotation of an object is in the direction of motion of the hands of a clock, it
is called clockwise rotation.
(ii) Anticlockwise: If an object rotates in the direction of motion opposite to that of the hands
of a clock, it is called anticlockwise rotation.

Anticlockwise
rotation O

O

Clockwise
rotation

A clock Wheel of a bicycle A windmill

Angle of rotation
The minimum angle through which an object or a figure rotates about a fixed point to coincide with
itself is known as the angle of rotation. An object is said to take a full turn if it rotates by 360°.
A half-turn means a rotation by 180° and a quarter-turn means a rotation by 90°.
Rotational symmetry

A figure is said to have rotational symmetry if it fits into itself more than once during a full turn,
i.e., rotation through 360°.
Let’s consider three blades of a fan marked A, B and C as shown in Fig. 1. Now, rotate the fan
about point O in clockwise direction. When the fan is rotated by 120° (i.e., 1/3 of 360°) the blade A
takes the position of blade B, blade B takes the position of blade C and blade C takes the position
of blade A (as shown in Fig. 2). We observe that Fig. 2 looks exactly the same as the original Fig. 1.
One more rotation through 120° brings the blade to a new position as shown in Fig. 3. Finally
after a third rotation by 120°, the blades of the fan come back to their original position.

A C B A

120°
120°
O O O O O
C B A C B
B 120° A C

Fig. 1 Fig. 2 Fig. 3 Fig. 4
Thus in a full turn, there are precisely three positions (on rotation through the angles 120°, 240°
and 360°) when the fan looks exactly the same. Because of this, one can claim that a fan has a
rotational symmetry of order 3. Now, we give a formal definition of order of rotational symmetry.

217