Page 220 - Start Up Mathematics_7

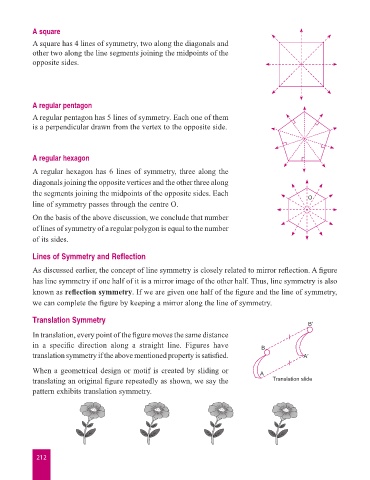
A square
A square has 4 lines of symmetry, two along the diagonals and
other two along the line segments joining the midpoints of the
opposite sides.

A regular pentagon
A regular pentagon has 5 lines of symmetry. Each one of them
is a perpendicular drawn from the vertex to the opposite side.

A regular hexagon
A regular hexagon has 6 lines of symmetry, three along the
diagonals joining the opposite vertices and the other three along

the segments joining the midpoints of the opposite sides. Each O
line of symmetry passes through the centre O.

On the basis of the above discussion, we conclude that number
of lines of symmetry of a regular polygon is equal to the number
of its sides.

Lines of Symmetry and Reflection

As discussed earlier, the concept of line symmetry is closely related to mirror reflection. A figure
has line symmetry if one half of it is a mirror image of the other half. Thus, line symmetry is also
known as reflection symmetry. If we are given one half of the figure and the line of symmetry,
we can complete the figure by keeping a mirror along the line of symmetry.

Translation Symmetry B′

In translation, every point of the figure moves the same distance
in a specific direction along a straight line. Figures have B
translation symmetry if the above mentioned property is satisfied. A′

When a geometrical design or motif is created by sliding or A

translating an original figure repeatedly as shown, we say the Translation slide
pattern exhibits translation symmetry.

212