Page 233 - Start Up Mathematics_7

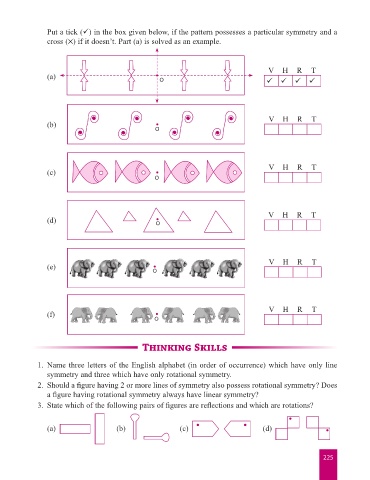
Put a tick () in the box given below, if the pattern possesses a particular symmetry and a
cross (✕) if it doesn’t. Part (a) is solved as an example.

V H R T
(a) O    

V H R T
(b)
O

V H R T
(c)
O

V H R T
(d) O

V H R T
(e)
O

V H R T
(f)
O

Thinking Skills

1. Name three letters of the English alphabet (in order of occurrence) which have only line
symmetry and three which have only rotational symmetry.
2. Should a figure having 2 or more lines of symmetry also possess rotational symmetry? Does
a figure having rotational symmetry always have linear symmetry?
3. State which of the following pairs of figures are reflections and which are rotations?

(a) (b) (c) (d)

225