Page 171 - Start Up Mathematics_7

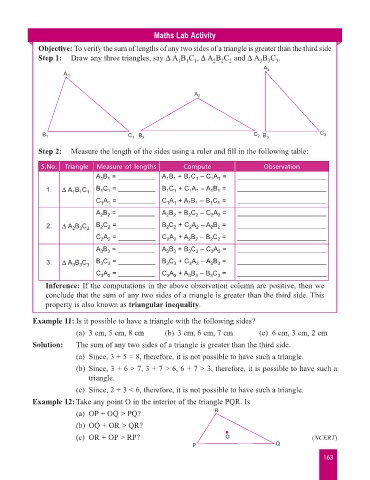
Maths Lab Activity

Objective: To verify the sum of lengths of any two sides of a triangle is greater than the third side
Step 1: Draw any three triangles, say ∆ A B C , ∆ A B C and ∆ A B C .
2 2 2
1 1 1
3 3 3
A 3
A 1

A 2

B 1 C 1 B 2 C 2 B 3 C 3

Step 2: Measure the length of the sides using a ruler and fill in the following table:

S.No. Triangle Measure of lengths Compute Observation
A B = __________ A B + B C – C A = ________________________
1 1
1
1 1
1 1
1
1. ∆ A B C 1 B C = __________ B C + C A – A B = ________________________
1
1
1
1 1
1
1 1
1 1
C A = __________ C A + A B – B C = ________________________
1 1
1
1 1
1 1
1
A B = __________ A B + B C – C A = ________________________
2 2
2 2
2 2
2
2
2. ∆ A B C 2 B C = __________ B C + C A – A B = ________________________
2 2
2
2 2
2
2
2
2 2
C A = __________ C A + A B – B C = ________________________
2
2 2
2 2
2
2 2
A B = __________ A B + B C – C A = ________________________
3 3
3
3 3
3
3 3
3. ∆ A B C 3 B C = __________ B C + C A – A B = ________________________
3 3
3 3
3
3
3
3
3 3
C A = __________ C A + A B – B C = ________________________
3
3 3
3 3
3
3 3
Inference: If the computations in the above observation column are positive, then we
conclude that the sum of any two sides of a triangle is greater than the third side. This
property is also known as triangular inequality.
Example 11: Is it possible to have a triangle with the following sides?
(a) 3 cm, 5 cm, 8 cm (b) 3 cm, 6 cm, 7 cm (c) 6 cm, 3 cm, 2 cm
Solution: The sum of any two sides of a triangle is greater than the third side.
(a) Since, 3 + 5 = 8, therefore, it is not possible to have such a triangle.
(b) Since, 3 + 6 > 7, 3 + 7 > 6, 6 + 7 > 3, therefore, it is possible to have such a
triangle.
(c) Since, 2 + 3 < 6, therefore, it is not possible to have such a triangle.
Example 12: Take any point O in the interior of the triangle PQR. Is
(a) OP + OQ > PQ? R
(b) OQ + OR > QR?
(c) OR + OP > RP? O (NCERT)
P Q
163