Page 169 - Start Up Mathematics_7

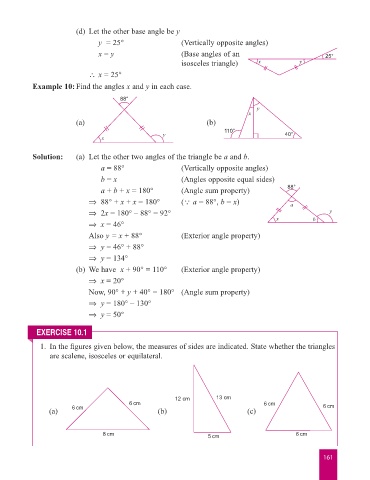
(d) Let the other base angle be y

y = 25° (Vertically opposite angles)
x = y (Base angles of an 25°
isosceles triangle) x y
∴ x = 25°

Example 10: Find the angles x and y in each case.

88°
y
x
(a) (b)
110°
y 40°
x

Solution: (a) Let the other two angles of the triangle be a and b.

a = 88° (Vertically opposite angles)
b = x (Angles opposite equal sides)
a + b + x = 180° (Angle sum property) 88°
⇒ 88° + x + x = 180° ( a = 88°, b = x) a

⇒ 2x = 180° – 88° = 92° y
⇒ x = 46° x b

Also y = x + 88° (Exterior angle property)
⇒ y = 46° + 88°
⇒ y = 134°
(b) We have x + 90° = 110° (Exterior angle property)

⇒ x = 20°
Now, 90° + y + 40° = 180° (Angle sum property)
⇒ y = 180° – 130°

⇒ y = 50°

EXERCISE 10.1
1. In the figures given below, the measures of sides are indicated. State whether the triangles
are scalene, isosceles or equilateral.

12 cm 13 cm
6 cm 6 cm 6 cm
(a) 6 cm (b) (c)

8 cm 5 cm 6 cm

161