Page 176 - Start Up Mathematics_7

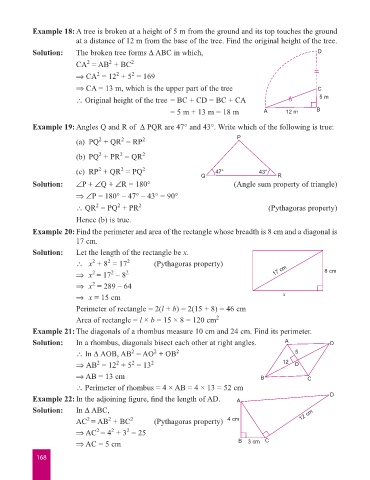
Example 18: A tree is broken at a height of 5 m from the ground and its top touches the ground
at a distance of 12 m from the base of the tree. Find the original height of the tree.

Solution: The broken tree forms ∆ ABC in which, D
2
2
CA = AB + BC 2
2
2
2
⇒ CA = 12 + 5 = 169
⇒ CA = 13 m, which is the upper part of the tree C
∴ Original height of the tree = BC + CD = BC + CA 5 m
= 5 m + 13 m = 18 m A 12 m B

Example 19: Angles Q and R of ∆ PQR are 47° and 43°. Write which of the following is true:

2
2
(a) PQ + QR = RP 2 P
2
2
(b) PQ + PR = QR 2
2
2
(c) RP + QR = PQ 2 47° 43°
Q R
Solution: ∠P + ∠Q + ∠R = 180° (Angle sum property of triangle)
⇒ ∠P = 180° – 47° – 43° = 90°

2
2
2
∴ QR = PQ + PR (Pythagoras property)
Hence (b) is true.
Example 20: Find the perimeter and area of the rectangle whose breadth is 8 cm and a diagonal is
17 cm.
Solution: Let the length of the rectangle be x.
2
2
∴ x + 8 = 17 2 (Pythagoras property)
2
2
⇒ x = 17 – 8 2 17 cm 8 cm
2
⇒ x = 289 – 64
⇒ x = 15 cm x
Perimeter of rectangle = 2(l + b) = 2(15 + 8) = 46 cm
Area of rectangle = l × b = 15 × 8 = 120 cm 2
Example 21: The diagonals of a rhombus measure 10 cm and 24 cm. Find its perimeter.

Solution: In a rhombus, diagonals bisect each other at right angles. A D
2
2
∴ In ∆ AOB, AB = AO + OB 2 5
2
2
2
⇒ AB = 12 + 5 = 13 2 12 O
⇒ AB = 13 cm B C
∴ Perimeter of rhombus = 4 × AB = 4 × 13 = 52 cm
Example 22: In the adjoining figure, find the length of AD. A D

Solution: In ∆ ABC, 12 cm
2
2
AC = AB + BC 2 (Pythagoras property) 4 cm
2
2
2
⇒ AC = 4 + 3 = 25
⇒ AC = 5 cm B 3 cm C
168