Page 95 - Start Up Mathematics_6

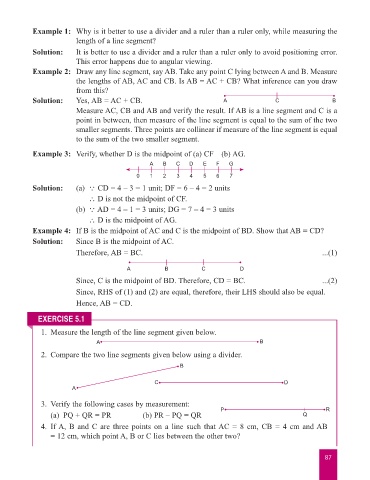
Example 1: Why is it better to use a divider and a ruler than a ruler only, while measuring the
length of a line segment?

Solution: It is better to use a divider and a ruler than a ruler only to avoid positioning error.
This error happens due to angular viewing.
Example 2: Draw any line segment, say AB. Take any point C lying between A and B. Measure
the lengths of AB, AC and CB. Is AB = AC + CB? What inference can you draw
from this?
Solution: Yes, AB = AC + CB. A C B
Measure AC, CB and AB and verify the result. If AB is a line segment and C is a
point in between, then measure of the line segment is equal to the sum of the two
smaller segments. Three points are collinear if measure of the line segment is equal
to the sum of the two smaller segment.

Example 3: Verify, whether D is the midpoint of (a) CF (b) AG.
A B C D E F G

0 1 2 3 4 5 6 7
Solution: (a)  CD = 4 – 3 = 1 unit; DF = 6 – 4 = 2 units
∴ D is not the midpoint of CF.
(b)  AD = 4 – 1 = 3 units; DG = 7 – 4 = 3 units
∴ D is the midpoint of AG.
Example 4: If B is the midpoint of AC and C is the midpoint of BD. Show that AB = CD?

Solution: Since B is the midpoint of AC.
Therefore, AB = BC. ...(1)

A B C D
Since, C is the midpoint of BD. Therefore, CD = BC. ...(2)
Since, RHS of (1) and (2) are equal, therefore, their LHS should also be equal.
Hence, AB = CD.

EXERCISE 5.1
1. Measure the length of the line segment given below.

A B
2. Compare the two line segments given below using a divider.

B

C D
A

3. Verify the following cases by measurement:
(a) PQ + QR = PR (b) PR – PQ = QR P Q R

4. If A, B and C are three points on a line such that AC = 8 cm, CB = 4 cm and AB
= 12 cm, which point A, B or C lies between the other two?

87