Page 57 - Start Up Mathematics_5

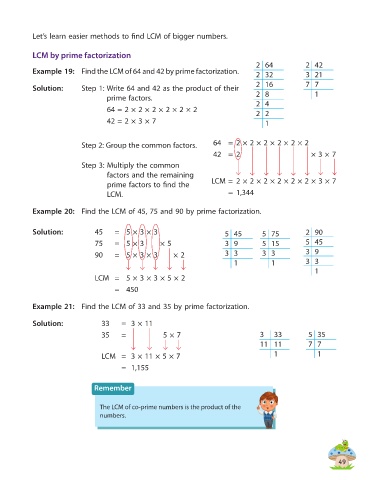
Let’s learn easier methods to find LCM of bigger numbers.

LCM by prime factorization
2 64 2 42
Example 19: Find the LCM of 64 and 42 by prime factorization. 2 32 3 21

Solution: Step 1: Write 64 and 42 as the product of their 2 16 7 7
prime factors. 2 8 1

64 = 2 × 2 × 2 × 2 × 2 × 2 2 4
2 2
42 = 2 × 3 × 7 1

Step 2: Group the common factors. 64 = 2 × 2 × 2 × 2 × 2 × 2
42 = 2 × 3 × 7
Step 3: Multiply the common
factors and the remaining
prime factors to find the LCM = 2 × 2 × 2 × 2 × 2 × 2 × 3 × 7
LCM. = 1,344

Example 20: Find the LCM of 45, 75 and 90 by prime factorization.

Solution: 45 = 5 × 3 × 3 5 45 5 75 2 90
75 = 5 × 3 × 5 3 9 5 15 5 45

90 = 5 × 3 × 3 × 2 3 3 3 3 3 9
1 1 3 3
1
LCM = 5 × 3 × 3 × 5 × 2

= 450

Example 21: Find the LCM of 33 and 35 by prime factorization.

Solution: 33 = 3 × 11
35 = 5 × 7 3 33 5 35
11 11 7 7
LCM = 3 × 11 × 5 × 7 1 1

= 1,155

Remember

The LCM of co-prime numbers is the product of the
numbers.

49