Page 55 - Start Up Mathematics_5

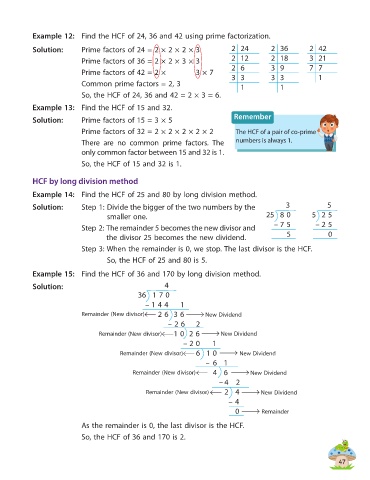
Example 12: Find the HCF of 24, 36 and 42 using prime factorization.

Solution: Prime factors of 24 = 2 × 2 × 2 × 3 2 24 2 36 2 42

Prime factors of 36 = 2 × 2 × 3 × 3 2 12 2 18 3 21
Prime factors of 42 = 2 × 3 × 7 2 6 3 9 7 7
3 3
1
3 3
Common prime factors = 2, 3 1 1
So, the HCF of 24, 36 and 42 = 2 × 3 = 6.
Example 13: Find the HCF of 15 and 32.

Solution: Prime factors of 15 = 3 × 5 Remember
Prime factors of 32 = 2 × 2 × 2 × 2 × 2 The HCF of a pair of co-prime
There are no common prime factors. The numbers is always 1.
only common factor between 15 and 32 is 1.

So, the HCF of 15 and 32 is 1.

HCF by long division method

Example 14: Find the HCF of 25 and 80 by long division method.

Solution: Step 1: Divide the bigger of the two numbers by the 3 5
smaller one. 25 8 0 5 2 5
Step 2: The remainder 5 becomes the new divisor and – 7 5 – 2 5
the divisor 25 becomes the new dividend. 5 0
Step 3: When the remainder is 0, we stop. The last divisor is the HCF.

So, the HCF of 25 and 80 is 5.

Example 15: Find the HCF of 36 and 170 by long division method.
Solution: 4
36 1 7 0
– 1 4 4 1

Remainder (New divisor) 2 6 3 6 New Dividend
– 2 6 2

Remainder (New divisor) 1 0 2 6 New Dividend
– 2 0 1

Remainder (New divisor) 6 1 0 New Dividend
– 6 1
Remainder (New divisor) 4 6 New Dividend
– 4 2
2 4 New Dividend
Remainder (New divisor)
– 4
0 Remainder

As the remainder is 0, the last divisor is the HCF.
So, the HCF of 36 and 170 is 2.

47