Page 52 - Start Up Mathematics_5

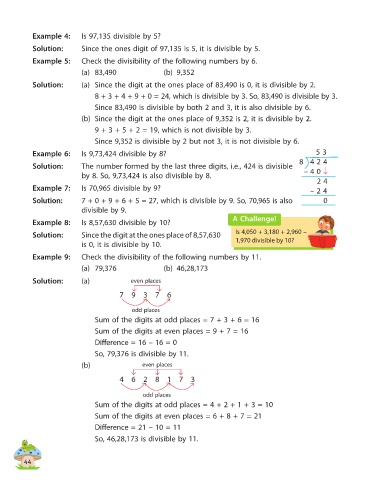
Example 4: Is 97,135 divisible by 5?

Solution: Since the ones digit of 97,135 is 5, it is divisible by 5.

Example 5: Check the divisibility of the following numbers by 6.
(a) 83,490 (b) 9,352
Solution: (a) Since the digit at the ones place of 83,490 is 0, it is divisible by 2.

8 + 3 + 4 + 9 + 0 = 24, which is divisible by 3. So, 83,490 is divisible by 3.
Since 83,490 is divisible by both 2 and 3, it is also divisible by 6.

(b) Since the digit at the ones place of 9,352 is 2, it is divisible by 2.
9 + 3 + 5 + 2 = 19, which is not divisible by 3.
Since 9,352 is divisible by 2 but not 3, it is not divisible by 6.

Example 6: Is 9,73,424 divisible by 8? 5 3
8 4 2 4
Solution: The number formed by the last three digits, i.e., 424 is divisible – 4 0 ↓
by 8. So, 9,73,424 is also divisible by 8.
2 4
Example 7: Is 70,965 divisible by 9? – 2 4
Solution: 7 + 0 + 9 + 6 + 5 = 27, which is divisible by 9. So, 70,965 is also 0
divisible by 9.

Example 8: Is 8,57,630 divisible by 10? A Challenge!

Solution: Since the digit at the ones place of 8,57,630 Is 4,050 + 3,180 + 2,960 –
is 0, it is divisible by 10. 1,970 divisible by 10?

Example 9: Check the divisibility of the following numbers by 11.
(a) 79,376 (b) 46,28,173

Solution: (a) even places

7 9 3 7 6

odd places
Sum of the digits at odd places = 7 + 3 + 6 = 16
Sum of the digits at even places = 9 + 7 = 16

Difference = 16 – 16 = 0
So, 79,376 is divisible by 11.
(b) even places

4 6 2 8 1 7 3

odd places
Sum of the digits at odd places = 4 + 2 + 1 + 3 = 10

Sum of the digits at even places = 6 + 8 + 7 = 21
Difference = 21 – 10 = 11

So, 46,28,173 is divisible by 11.

44