Page 211 - ICSE Math 8

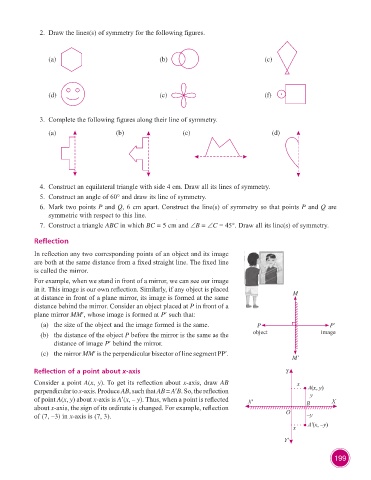
2. Draw the lines(s) of symmetry for the following figures.

(a) (b) (c)

(d) (e) (f)

3. Complete the following figures along their line of symmetry.

(a) (b) (c) (d)

4. Construct an equilateral triangle with side 4 cm. Draw all its lines of symmetry.
5. Construct an angle of 60° and draw its line of symmetry.
6. Mark two points P and Q, 6 cm apart. Construct the line(s) of symmetry so that points P and Q are
symmetric with respect to this line.
7. Construct a triangle ABC in which BC = 5 cm and ∠B = ∠C = 45°. Draw all its line(s) of symmetry.

Reflection
In reflection any two corresponding points of an object and its image
are both at the same distance from a fixed straight line. The fixed line
is called the mirror.
For example, when we stand in front of a mirror, we can see our image
in it. This image is our own reflection. Similarly, if any object is placed M
at distance in front of a plane mirror, its image is formed at the same
distance behind the mirror. Consider an object placed at P in front of a
plane mirror MM′, whose image is formed at P′ such that:
(a) the size of the object and the image formed is the same. P P'
(b) the distance of the object P before the mirror is the same as the object image
distance of image P′ behind the mirror.
(c) the mirror MM′ is the perpendicular bisector of line segment PP′.
M'

Reflection of a point about x-axis
Consider a point A(x, y). To get its reflection about x-axis, draw AB
perpendicular to x-axis. Produce AB, such that AB = A′B. So, the reflection
of point A(x, y) about x-axis is A′(x, – y). Thus, when a point is reflected
about x-axis, the sign of its ordinate is changed. For example, reflection
of (7, –3) in x-axis is (7, 3).

199