Page 184 - ICSE Math 8

The parallelogram is a rectangle.
So, quadrilateral ABCD will be a parallelogram only if it is a rectangle otherwise not.
(b) AB = DC = 9 cm, AD = 5 cm and BC = 5.5 cm

No, quadrilateral ABCD cannot be a parallelogram with these conditions, because
AD π BC (required condition of a parallelogram is that opposite sides should be equal).

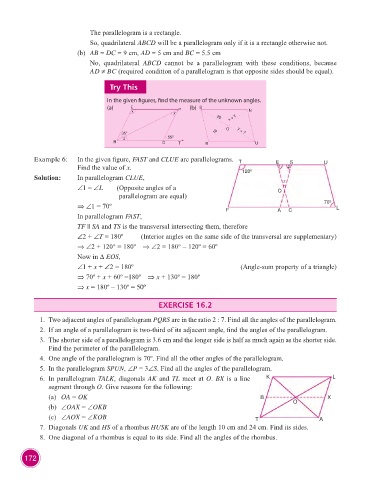
Try This
In the given figures, find the measure of the unknown angles.
(a) E P (b) S
x y N
20 x + y

35º 16 O y + 7
z 55º
R O T R U

Example 6: In the given figure, FAST and CLUE are parallelograms. T E S U
Find the value of x. 120º 1 2
Solution: In parallelogram CLUE, x
–1 = –L ( Opposite angles of a O
parallelogram are equal)
fi –1 = 70º 70º L
F A C
In parallelogram FAST,
TF || SA and TS is the transversal intersecting them, therefore

–2 + –T = 180º (Interior angles on the same side of the transversal are supplementary)
fi –2 + 120° = 180º fi –2 = 180° – 120º = 60º
Now in D EOS,
–1 + x + –2 = 180º (Angle-sum property of a triangle)
fi 70º + x + 60º =180º fi x + 130º = 180º
fi x = 180º – 130º = 50º

EXERCISE 16.2

1. Two adjacent angles of parallelogram PQRS are in the ratio 2 : 7. Find all the angles of the parallelogram.
2. If an angle of a parallelogram is two-third of its adjacent angle, find the angles of the parallelogram.
3. The shorter side of a parallelogram is 3.6 cm and the longer side is half as much again as the shorter side.
Find the perimeter of the parallelogram.
4. One angle of the parallelogram is 70º. Find all the other angles of the parallelogram.
5. In the parallelogram SPUN, ∠P = 3∠S. Find all the angles of the parallelogram.
6. In parallelogram TALK, diagonals AK and TL meet at O. BX is a line K L
segment through O. Give reasons for the following:
(a) OA = OK B O X
(b) ∠OAX = ∠OKB
(c) ∠AOX = ∠KOB T A
7. Diagonals UK and HS of a rhombus HUSK are of the length 10 cm and 24 cm. Find its sides.
8. One diagonal of a rhombus is equal to its side. Find all the angles of the rhombus.

172