Page 100 - ICSE Math 8

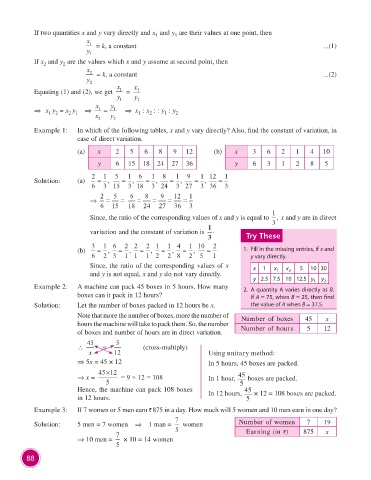
If two quantities x and y vary directly and x and y are their values at one point, then
1
1
x
1 = k, a constant ...(1)
y 1
If x and y are the values which x and y assume at second point, then
2
2
x 2 = k, a constant ...(2)
y 2

Equating (1) and (2), we get x 1 = x 2
y 1 y 2
x y
fi x y = x y fi x 1 2 = y 1 2 fi x : x : : y : y 2
1 2
2 1
1
2
1
Example 1: In which of the following tables, x and y vary directly? Also, find the constant of variation, in
case of direct variation.
(a) x 2 5 6 8 9 12 (b) x 3 6 2 1 4 10
y 6 15 18 24 27 36 y 6 3 1 2 8 5

2 1 5 1 6 1 8 1 9 1 12 1
Solution: (a) = , = , = , = , = , =
6 3 15 3 18 3 24 3 27 3 36 3
2 5 6 8 9 12 1
fi = = = = = =
6 15 18 24 27 36 3
1
Since, the ratio of the corresponding values of x and y is equal to , x and y are in direct
1 3
variation and the constant of variation is .
3 Try These
3 1 6 2 2 2 1 1 4 1 10 2
(b) = , = , = , = , = , = 1. Fill in the missing entries, if x and
6 2 3 1 1 1 2 2 8 2 5 1 y vary directly.
Since, the ratio of the corresponding values of x x 1 x x 5 10 30
and y is not equal, x and y do not vary directly. 1 2
y 2.5 7.5 10 12.5 y 1 y 2
Example 2: A machine can pack 45 boxes in 5 hours. How many 2. A quantity A varies directly as B.
boxes can it pack in 12 hours? If A = 75, when B = 25, then find
Solution: Let the number of boxes packed in 12 hours be x. the value of A when B = 37.5.
Note that more the number of boxes, more the number of Number of boxes 45 x
hours the machine will take to pack them. So, the number
of boxes and number of hours are in direct variation. Number of hours 5 12

45 5
\ = (cross-multiply)
x 12 Using unitary method:
fi 5x = 45 × 12 In 5 hours, 45 boxes are packed.
45 12×
fi x = = 9 × 12 = 108 In 1 hour, 45 boxes are packed.
5 5
Hence, the machine can pack 108 boxes In 12 hours, 45 × 12 = 108 boxes are packed.
in 12 hours. 5

Example 3: If 7 women or 5 men earn ` 875 in a day. How much will 5 women and 10 men earn in one day?
7 Number of women
Solution: 5 men = 7 women fi 1 man = 5 women 7 19
7 Earning (in `) 875 x
fi 10 men = × 10 = 14 women
5

88