Page 102 - ICSE Math 8

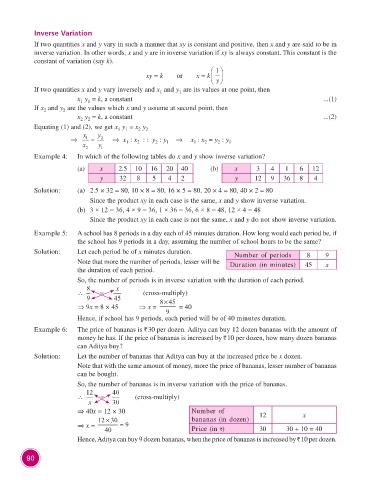
Inverse Variation

If two quantities x and y vary in such a manner that xy is constant and positive, then x and y are said to be in
inverse variation. In other words, x and y are in inverse variation if xy is always constant. This constant is the
constant of variation (say k).
 1
xy = k or x = k  

y
If two quantities x and y vary inversely and x and y are its values at one point, then
1
1
x y = k, a constant ...(1)
1
1
If x and y are the values which x and y assume at second point, then
2
2
x y = k, a constant ...(2)
2
2
Equating (1) and (2), we get x y = x y 2
1
1
2
x y
fi 1 = 2 fi x : x : : y : y fi x : x = y : y 1
2
1
1
1
2
2
2
x 2 y 1
Example 4: In which of the following tables do x and y show inverse variation?
(a) x 2.5 10 16 20 40 (b) x 3 4 1 6 12
y 32 8 5 4 2 y 12 9 36 8 4
Solution: (a) 2.5 × 32 = 80, 10 × 8 = 80, 16 × 5 = 80, 20 × 4 = 80, 40 × 2 = 80
Since the product xy in each case is the same, x and y show inverse variation.
(b) 3 × 12 = 36, 4 × 9 = 36, 1 × 36 = 36, 6 × 8 = 48, 12 × 4 = 48
Since the product xy in each case is not the same, x and y do not show inverse variation.
Example 5: A school has 8 periods in a day each of 45 minutes duration. How long would each period be, if
the school has 9 periods in a day, assuming the number of school hours to be the same?
Solution: Let each period be of x minutes duration. Number of periods 8 9
Note that more the number of periods, lesser will be Duration (in minutes) 45 x
the duration of each period.
So, the number of periods is in inverse variation with the duration of each period.
8 x
\ = (cross-multiply)
9 45 845×
fi 9x = 8 × 45 fi x = 9 = 40

Hence, if school has 9 periods, each period will be of 40 minutes duration.
Example 6: The price of bananas is ` 30 per dozen. Aditya can buy 12 dozen bananas with the amount of
money he has. If the price of bananas is increased by ` 10 per dozen, how many dozen bananas
can Aditya buy?

Solution: Let the number of bananas that Aditya can buy at the increased price be x dozen.
Note that with the same amount of money, more the price of bananas, lesser number of bananas
can be bought.
So, the number of bananas is in inverse variation with the price of bananas.
12 40
\ = (cross-multiply)
x 30
fi 40x = 12 × 30 Number of 12 x
12 30× bananas (in dozen)
fi x = = 9
40 Price (in `) 30 30 + 10 = 40
Hence, Aditya can buy 9 dozen bananas, when the price of bananas is increased by ` 10 per dozen.

90