Page 143 - Start Up Mathematics_8 (Non CCE)

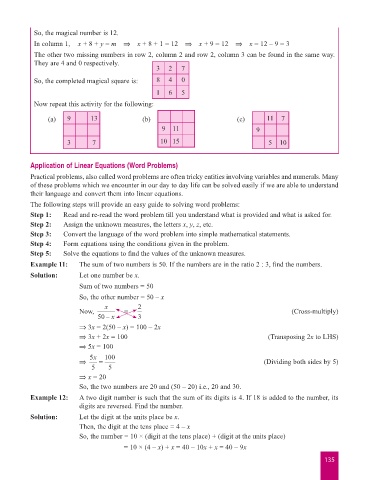
So, the magical number is 12.
In column 1, x + 8 + y = m fi x + 8 + 1 = 12 fi x + 9 = 12 fi x = 12 – 9 = 3

The other two missing numbers in row 2, column 2 and row 2, column 3 can be found in the same way.
They are 4 and 0 respectively.
3 2 7

So, the completed magical square is: 8 4 0
1 6 5

Now repeat this activity for the following:

(a) 9 13 (b) (c) 11 7
9 11 9

3 7 10 15 5 10

Application of Linear Equations (Word Problems)
Practical problems, also called word problems are often tricky entities involving variables and numerals. Many
of these problems which we encounter in our day to day life can be solved easily if we are able to understand
their language and convert them into linear equations.
The following steps will provide an easy guide to solving word problems:
Step 1: Read and re-read the word problem till you understand what is provided and what is asked for.
Step 2: Assign the unknown measures, the letters x, y, z, etc.
Step 3: Convert the language of the word problem into simple mathematical statements.
Step 4: Form equations using the conditions given in the problem.
Step 5: Solve the equations to find the values of the unknown measures.
Example 11: The sum of two numbers is 50. If the numbers are in the ratio 2 : 3, find the numbers.
Solution: Let one number be x.

Sum of two numbers = 50
So, the other number = 50 – x
x 2
Now, = (Cross-multiply)
50 - x 3
fi 3x = 2(50 – x) = 100 – 2x
fi 3x + 2x = 100 (Transposing 2x to LHS)
fi 5x = 100
5x 100
fi = (Dividing both sides by 5)
5 5
fi x = 20
So, the two numbers are 20 and (50 – 20) i.e., 20 and 30.

Example 12: A two digit number is such that the sum of its digits is 4. If 18 is added to the number, its
digits are reversed. Find the number.

Solution: Let the digit at the units place be x.
Then, the digit at the tens place = 4 – x
So, the number = 10 × (digit at the tens place) + (digit at the units place)

= 10 × (4 – x) + x = 40 – 10x + x = 40 – 9x
135