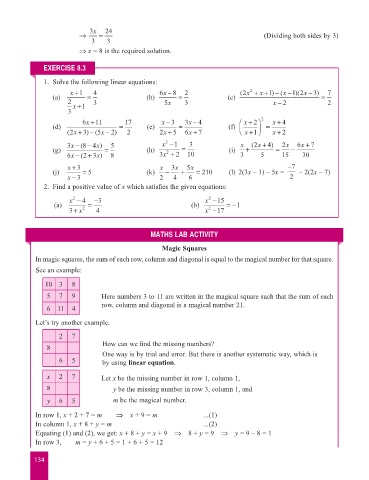
Page 142 - Start Up Mathematics_8 (Non CCE)

3x 24
fi = (Dividing both sides by 3)
3 3
fi x = 8 is the required solution.

EXERCISE 8.3

1. Solve the following linear equations:
2
x
x +1 4 6x - 8 2 (2x ++ 1 ) (x- - 12x - ) 3 7
)(
(a) = (b) = (c) =
2 x +1 3 5x 3 x - 2 2
3
6x + 11 17 x - 3 3 x - 4 Ê x + ˆ 2 2 x + 4
(d) = (e) = (f) Á ˜ =
( 2x + 3 -) ( 5x - 2) 2 2 x + 5 6 x + 7 Ë x + ¯ 1 x + 2
2
3x - ( 8 4x- ) 5 x - 1 3 x 2 ( x 4+ ) 2 x 6 x 7+
(g) = (h) 2 = (i) + = -
+
6x - ( 2 3x) 8 x 3 + 2 10 3 5 15 30
x + 3 x x 3 x 5 -7
(j) = 5 (k) - + = 210 (l) 2(3x – 1) – 5x = – 2(2x – 7)
x - 3 2 4 6 2
2. Find a positive value of x which satisfies the given equations:

x - 4 - 3 x - 15
2
2
(a) = (b) =- 1
2
3+ x 2 4 x - 17
MATHS LAB ACTIVITY

Magic Squares
In magic squares, the sum of each row, column and diagonal is equal to the magical number for that square.

See an example:
10 3 8

5 7 9 Here numbers 3 to 11 are written in the magical square such that the sum of each
6 11 4 row, column and diagonal is a magical number 21.

Let’s try another example.

2 7

8 How can we find the missing numbers?
One way is by trial and error. But there is another systematic way, which is
6 5 by using linear equation.

x 2 7 Let x be the missing number in row 1, column 1,

8 y be the missing number in row 3, column 1, and
y 6 5 m be the magical number.

In row 1, x + 2 + 7 = m fi x + 9 = m ...(1)
In column 1, x + 8 + y = m ...(2)
Equating (1) and (2), we get: x + 8 + y = x + 9 fi 8 + y = 9 fi y = 9 – 8 = 1
In row 3, m = y + 6 + 5 = 1 + 6 + 5 = 12

134