Page 138 - Start Up Mathematics_8 (Non CCE)

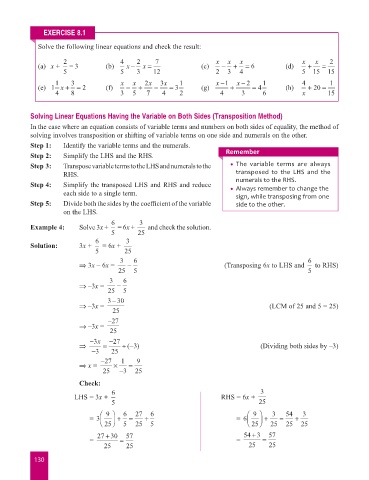
EXERCISE 8.1

Solve the following linear equations and check the result:

2 4 2 7 x x x x x 2
(a) x + =3 (b) x - x = (c) - + = 6 (d) + =
5 5 3 12 2 3 4 5 15 15
1 3 x x 2 x x 3 1 x -1 x - 2 1 4 1
(e) 1 x + = 2 (f) - + - = 3 (g) + = 4 (h) + 20 =
4 8 3 5 7 4 2 4 3 6 x 15

Solving Linear Equations Having the Variable on Both Sides (Transposition Method)
In the case where an equation consists of variable terms and numbers on both sides of equality, the method of
solving involves transposition or shifting of variable terms on one side and numerals on the other.
Step 1: Identify the variable terms and the numerals.
Step 2: Simplify the LHS and the RHS. Remember
Step 3: Transpose variable terms to the LHS and numerals to the • The variable terms are always
RHS. transposed to the LHS and the
numerals to the RHS.
Step 4: Simplify the transposed LHS and RHS and reduce • Always remember to change the
each side to a single term. sign, while transposing from one
Step 5: Divide both the sides by the coefficient of the variable side to the other.
on the LHS.
6 3
Example 4: Solve 3x + = 6x + and check the solution.
5 25
6 3
Solution: 3x + = 6x +
5 25
3 6 6
fi 3x – 6x = - (Transposing 6x to LHS and to RHS)
25 5 5
3 6
fi –3x = -
25 5
330-
fi –3x = (LCM of 25 and 5 = 25)
25
-27
fi –3x =
25
-3x -27
(
fi = ∏-3) (Dividing both sides by –3)
-3 25
-27 1 9
fi x = ¥ =
25 -3 25

Check:
6 3
LHS = 3x + RHS = 6x +
5 25

Ê 9 ˆ 6 27 6 Ê 9 ˆ 3 54 3
= 3 Á 25¯ + 5 = 25 + = 6 Á Ë 25¯ ˜ + 25 = 25 + 25
˜
Ë
5
= 27 30+ = 57 = 54 3+ = 57
25 25 25 25

130