Page 141 - ICSE Math 6

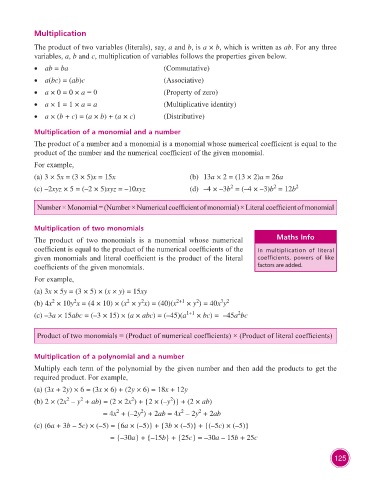
Multiplication

The product of two variables (literals), say, a and b, is a × b, which is written as ab. For any three
variables, a, b and c, multiplication of variables follows the properties given below.

• ab = ba (Commutative)
• a(bc) = (ab)c (Associative)

• a × 0 = 0 × a = 0 (Property of zero)
• a × 1 = 1 × a = a (Multiplicative identity)

• a × (b + c) = (a × b) + (a × c) (Distributive)

Multiplication of a monomial and a number
The product of a number and a monomial is a monomial whose numerical coefficient is equal to the
product of the number and the numerical coefficient of the given monomial.

For example,
(a) 3 × 5x = (3 × 5)x = 15x (b) 13a × 2 = (13 × 2)a = 26a
2
2
(c) –2xyz × 5 = (–2 × 5)xyz = –10xyz (d) –4 × –3b = (–4 × –3)b = 12b 2
Number × Monomial = (Number × Numerical coefficient of monomial) × Literal coefficient of monomial

Multiplication of two monomials
The product of two monomials is a monomial whose numerical Maths Info
coefficient is equal to the product of the numerical coefficients of the In multiplication of literal
given monomials and literal coefficient is the product of the literal coefficients, powers of like
coefficients of the given monomials. factors are added.
For example,

(a) 3x × 5y = (3 × 5) × (x × y) = 15xy
2
2
2
2
2
3 2
(b) 4x × 10y x = (4 × 10) × (x × y x) = (40)(x 2+1 × y ) = 40x y
2
(c) –3a × 15abc = (–3 × 15) × (a × abc) = (–45)(a 1+1 × bc) = –45a bc
Product of two monomials = (Product of numerical coefficients) × (Product of literal coefficients)

Multiplication of a polynomial and a number
Multiply each term of the polynomial by the given number and then add the products to get the
required product. For example,
(a) (3x + 2y) × 6 = (3x × 6) + (2y × 6) = 18x + 12y

2
2
2
2
(b) 2 × (2x – y + ab) = (2 × 2x ) + {2 × (–y )} + (2 × ab)
2
2
2
2
= 4x + (–2y ) + 2ab = 4x – 2y + 2ab
(c) (6a + 3b – 5c) × (–5) = {6a × (–5)} + {3b × (–5)} + {(–5c) × (–5)}
= {–30a} + {–15b} + {25c} = –30a – 15b + 25c
125