Page 24 - ICSE Math 5

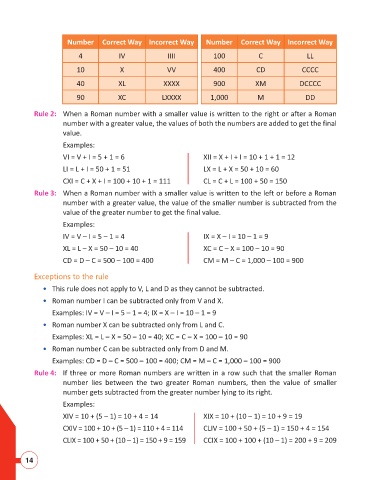
Number Correct Way Incorrect Way Number Correct Way Incorrect Way

4 IV IIII 100 C LL

10 X VV 400 CD CCCC

40 XL XXXX 900 XM DCCCC

90 XC LXXXX 1,000 M DD

Rule 2: When a Roman number with a smaller value is written to the right or after a Roman
number with a greater value, the values of both the numbers are added to get the final
value.

Examples:
VI = V + I = 5 + 1 = 6 XII = X + I + I = 10 + 1 + 1 = 12

LI = L + I = 50 + 1 = 51 LX = L + X = 50 + 10 = 60
CXI = C + X + I = 100 + 10 + 1 = 111 CL = C + L = 100 + 50 = 150

Rule 3: When a Roman number with a smaller value is written to the left or before a Roman
number with a greater value, the value of the smaller number is subtracted from the
value of the greater number to get the final value.
Examples:

IV = V – I = 5 – 1 = 4 IX = X – I = 10 – 1 = 9
XL = L – X = 50 – 10 = 40 XC = C – X = 100 – 10 = 90

CD = D – C = 500 – 100 = 400 CM = M – C = 1,000 – 100 = 900

Exceptions to the rule

• This rule does not apply to V, L and D as they cannot be subtracted.
• Roman number I can be subtracted only from V and X.

Examples: IV = V – I = 5 – 1 = 4; IX = X – I = 10 – 1 = 9
• Roman number X can be subtracted only from L and C.

Examples: XL = L – X = 50 – 10 = 40; XC = C – X = 100 – 10 = 90
• Roman number C can be subtracted only from D and M.

Examples: CD = D – C = 500 – 100 = 400; CM = M – C = 1,000 – 100 = 900
Rule 4: If three or more Roman numbers are written in a row such that the smaller Roman
number lies between the two greater Roman numbers, then the value of smaller
number gets subtracted from the greater number lying to its right.

Examples:
XIV = 10 + (5 – 1) = 10 + 4 = 14 XIX = 10 + (10 – 1) = 10 + 9 = 19

CXIV = 100 + 10 + (5 – 1) = 110 + 4 = 114 CLIV = 100 + 50 + (5 – 1) = 150 + 4 = 154
CLIX = 100 + 50 + (10 – 1) = 150 + 9 = 159 CCIX = 100 + 100 + (10 – 1) = 200 + 9 = 209

14