Page 88 - Start Up Mathematics_7

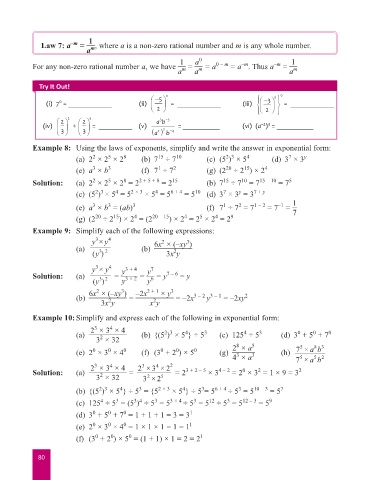
–m
Law 7: a = 1 , where a is a non-zero rational number and m is any whole number.
a m
1 a 0 1
–m
–m
For any non-zero rational number a, we have = = a 0 – m = a . Thus a =
a m a m a m
Try It Out!

5
− 
 5 0    −   0
3
0
(i) 7 = _____________ (ii)   = _____________ (iii)     = _____________
 2   2 
   
 2  2  2  5 ab − 3
3
–2 3
(iv)   ÷   = __________ (v) = ___________ (vi) (a ) = ___________
3
3
    a ( ) 2 b − 4
2
Example 8: Using the laws of exponents, simplify and write the answer in exponential form:
7
5
10
2
4
8
15
2 3
(a) 2 × 2 × 2 (b) 7 ÷ 7 (c) (5 ) × 5 (d) 3 × 3 y
1
2
20
15
3
3
(e) a × b (f) 7 ÷ 7 (g) (2 ÷ 2 ) × 2 4
8
15
2
5
10
Solution: (a) 2 × 2 × 2 = 2 2 + 5 + 8 = 2 15 (b) 7 ÷ 7 = 7 15 – 10 = 7 5
10
4
4
7
2 3
y
(c) (5 ) × 5 = 5 2 × 3 × 5 = 5 6 + 4 = 5 (d) 3 × 3 = 3 7 + y
3
3
3
1
–1
2
(e) a × b = (ab) (f) 7 ÷ 7 = 7 1 – 2 = 7 = 1
7
15
4
4
5
4
20
(g) (2 ÷ 2 ) × 2 = (2 20 – 15 ) × 2 = 2 × 2 = 2 9
Example 9: Simplify each of the following expressions:
× 6x × (–xy )
2
3
(a) (b)
2
( )y 3 2 3x y
× y 3 + 4 y 7
Solution: (a) = = = y 7 – 6 = y
()y 32 y 3 × 2 y 6
3
2
6x × (–xy ) –2x 2 + 1 × y 3
(b) = = –2x 3 – 2 3 – 1 = –2xy 2
y
2
2
3x y x y
Example 10: Simplify and express each of the following in exponential form:
4
3
2 × 3 × 4
4
2 3
4
0
0
(a) (b) {(5 ) × 5 } ÷ 5 3 (c) 125 ÷ 5 3 (d) 3 + 5 + 7 0
2
3 × 32
8
5
8 3
0
(e) 2 × 3 × 4 0 (f) (3 + 2 ) × 5 0 (g) 2 × a 5 (h) 7 × a b
0
0
0
3
4 × a 3 7 × a b
5
5 2
4
3
4
2 × 3 × 4 2 × 3 × 2
3
2
0
Solution: (a) = = 2 3 + 2 – 5 × 3 4 – 2 = 2 × 3 = 1 × 9 = 3 2
2
3 × 32 3 × 2 5
2
4
3
3
2 3
4
3
(b) {(5 ) × 5 } ÷ 5 = {5 2 × 3 × 5 } ÷ 5 = 5 6 + 4 ÷ 5 = 5 10 – 3 = 5 7
4
3
12
3 4
3
3
3
(c) 125 ÷ 5 = (5 ) ÷ 5 = 5 3 × 4 ÷ 5 = 5 ÷ 5 = 5 12 – 3 = 5 9
0
0
0
(d) 3 + 5 + 7 = 1 + 1 + 1 = 3 = 3 1
0
0
(e) 2 × 3 × 4 = 1 × 1 × 1 = 1 = 1 1
0
(f) (3 + 2 ) × 5 = (1 + 1) × 1 = 2 = 2 1
0
0
0
80