Page 86 - Start Up Mathematics_7
P. 86
4
2
5
3
11
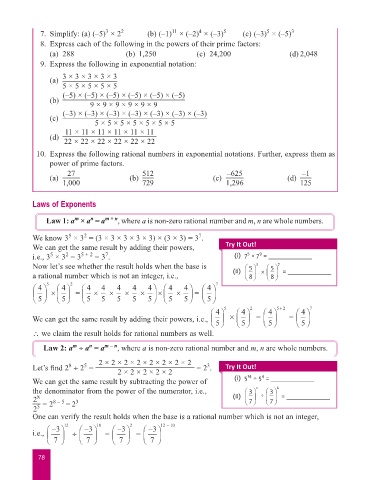
7. Simplify: (a) (–5) × 2 (b) (–1) × (–2) × (–3) 5 (c) (–3) × (–5) 3
8. Express each of the following in the powers of their prime factors:
(a) 288 (b) 1,250 (c) 24,200 (d) 2,048
9. Express the following in exponential notation:
(a) 3 × 3 × 3 × 3 × 3
5 × 5 × 5 × 5 × 5
(–5) × (–5) × (–5) × (–5) × (–5) × (–5)
(b)
9 × 9 × 9 × 9 × 9 × 9
(–3) × (–3) × (–3) × (–3) × (–3) × (–3) × (–3)
(c)
5 × 5 × 5 × 5 × 5 × 5 × 5
11 × 11 × 11 × 11 × 11 × 11
(d)
22 × 22 × 22 × 22 × 22 × 22
10. Express the following rational numbers in exponential notations. Further, express them as
power of prime factors.
27 512 –625 –1
(a) (b) (c) (d)
1,000 729 1,296 125
Laws of Exponents
m
n
Law 1: a × a = a m + n , where a is non-zero rational number and m, n are whole numbers.
7
2
5
We know 3 × 3 = (3 × 3 × 3 × 3 × 3) × (3 × 3) = 3 .
We can get the same result by adding their powers, Try It Out!
5
9
2
5
7
i.e., 3 × 3 = 3 5 + 2 = 3 . (i) 7 × 7 = _____________
Now let’s see whether the result holds when the base is 5 3 5 7
a rational number which is not an integer, i.e., (ii) × = _____________
8
8
4 5 4 2 4 4 4 4 4 4 4 4 7
×
× = × × × × × =
5 5 5 5 5 5 5 5 5 5
4 5 4 2 4 52+ 4 7
We can get the same result by adding their powers, i.e., × = =
5
5
5
5
∴ we claim the result holds for rational numbers as well.
n
m
Law 2: a ÷ a = a m – n , where a is non-zero rational number and m, n are whole numbers.
2 × 2 × 2 × 2 × 2 × 2 × 2 × 2
3
8
5
Let’s find 2 ÷ 2 = = 2 . Try It Out!
2 × 2 × 2 × 2 × 2
4
14
We can get the same result by subtracting the power of (i) 5 ÷ 5 = _____________
the denominator from the power of the numerator, i.e., 3 2 3 6
2 8 = 2 8 – 5 3 (ii) ÷ = _____________
7
7
= 2
2 5
One can verify the result holds when the base is a rational number which is not an integer,
−
−
−
−
i.e., 3 12 ÷ 3 10 = 3 2 = 3 12 − 10
7 7 7 7
78