Page 87 - Start Up Mathematics_7

mn
m n
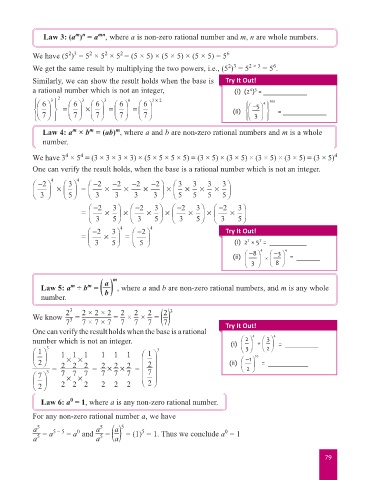
Law 3: (a ) = a , where a is non-zero rational number and m, n are whole numbers.
2 3
2
2
2
We have (5 ) = 5 × 5 × 5 = (5 × 5) × (5 × 5) × (5 × 5) = 5 6
2 3
6
We get the same result by multiplying the two powers, i.e., (5 ) = 5 2 × 3 = 5 .
Similarly, we can show the result holds when the base is Try It Out!
a rational number which is not an integer, (i) (2 ) = _____________
4 3
   6   2  6  3  6  3  6  6  6  3 × 2  4  100

3
5
    =   ×   =   =   (ii)     −   = _____________
 

   7    7   7   7   7     3  

m
m
m
Law 4: a × b = (ab) , where a and b are non-zero rational numbers and m is a whole
number.
4
4
We have 3 × 5 = (3 × 3 × 3 × 3) × (5 × 5 × 5 × 5) = (3 × 5) × (3 × 5) × (3 × 5) × (3 × 5) = (3 × 5) 4
One can verify the result holds, when the base is a rational number which is not an integer.
3
 2 4   4  −2 −2 −2 −   3 3 3 3 
2
− 
  ×   =  × × ×  ×  × × × 
 3     3 3 3 3   5 5 5 5 
5
 −2 3   −2 3   −2 3   −2 3 
=  ×  ×  ×  ×  ×  ×  × 
 3 5   3 5   3 5   3 5 
 −2 3  4  − 2  4 Try It Out!
=  ×  =  
7
7
 3 5 5   5  (i) 2 × 5 = ___________
− 
 8 4  3 4
− 
(ii)   ×   = _______
 3   8 
a m
m
m
Law 5: a ÷ b = b , where a and b are non-zero rational numbers, and m is any whole
number.
2 3 2 × 2 × 2 2 2 2 2 3
We know 3 = = × × =
7 7 × 7 × 7 7 7 7 7 Try It Out!
One can verify the result holds when the base is a rational
4
2 
3 
number which is not an integer. (i)    4 ÷    = __________
3
 1  3 1 1 1 1 1 1  1  3    2 
  ×× −  1 10

 2  = 2 2 2 = 2 × 2 × 2 =  2   (ii)    = ____________
 7  3 7 7 7 7 7 7  7   2 
××
  2 2 2 2 2 2  2 
 2 
0
Law 6: a = 1, where a is any non-zero rational number.
For any non-zero rational number a, we have
a 5 = a 5 – 5 = a and a 5 = a 5 = (1) = 1. Thus we conclude a = 1
5
0
0
a 5 a 5 a

79