Page 31 - Start Up Mathematics_7

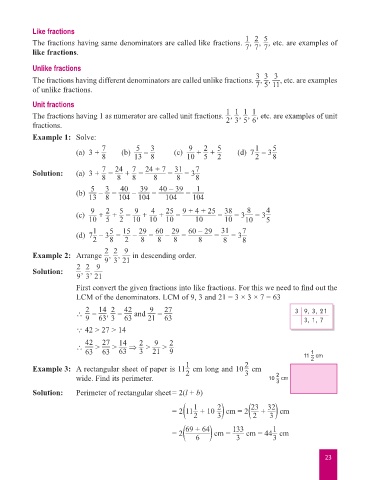
Like fractions
1 2 5
The fractions having same denominators are called like fractions. , , , etc. are examples of
7 7 7
like fractions.

Unlike fractions
3 3 3
The fractions having different denominators are called unlike fractions. , , , etc. are examples
7 5 11
of unlike fractions.
Unit fractions
1 1 1 1
The fractions having 1 as numerator are called unit fractions. , , , , etc. are examples of unit
2 3 5 6
fractions.
Example 1: Solve:

1
5
2
3
(a) 3 + 7 (b) 5 – (c) 9 + + (d) 7 – 3 5
8 13 8 10 5 2 2 8
7 24 7 24 + 7 31 7
Solution: (a) 3 + = + = = = 3
8 8 8 8 8 8
5 3 40 39 40 – 39 1
(b) – = – = =
13 8 104 104 104 104
9 2 5 9 4 25 9 + 4 + 25 38 8 4
(c) + + = + + = = = 3 = 3
10 5 2 10 10 10 10 10 10 5
1 5 15 29 60 29 60 – 29 31 7
(d) 7 – 3 = – = – = = = 3
2 8 2 8 8 8 8 8 8

2 2 9
Example 2: Arrange , , in descending order.
9 3 21
2 2 9
Solution: , ,
9 3 21
First convert the given fractions into like fractions. For this we need to find out the
LCM of the denominators. LCM of 9, 3 and 21 = 3 × 3 × 7 = 63

2 14 2 42 9 27 3 9, 3, 21
∴ = , = and =
9 63 3 63 21 63 3, 1, 7
 42 > 27 > 14

42 27 14 2 9 2
∴ > > ⇒ > >
63 63 63 3 21 9 1
11 cm
2
1 2
Example 3: A rectangular sheet of paper is 11 cm long and 10 cm
3
2
2
wide. Find its perimeter. 10 cm
3
Solution: Perimeter of rectangular sheet = 2(l + b)
1 2 23 32
= 2 11 + 10 cm = 2 + cm
2 3 2 3
69 + 64 133 1
= 2 cm = cm = 44 cm
6 3 3

2323