Page 117 - Start Up Mathematics_7

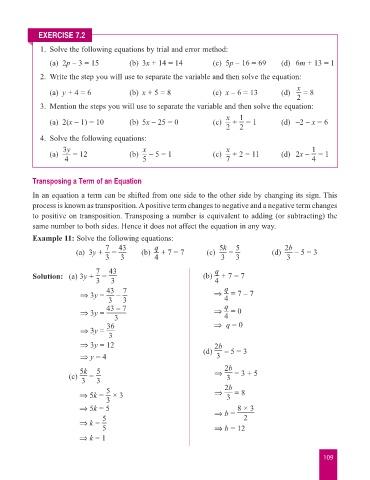
EXERCISE 7.2

1. Solve the following equations by trial and error method:

(a) 2p – 3 = 15 (b) 3x + 14 = 14 (c) 5p – 16 = 69 (d) 6m + 13 = 1

2. Write the step you will use to separate the variable and then solve the equation:
x
(a) y + 4 = 6 (b) x + 5 = 8 (c) x – 6 = 13 (d) = 8
2
3. Mention the steps you will use to separate the variable and then solve the equation:
x 1
(a) 2(x – 1) = 10 (b) 5x – 25 = 0 (c) + = 1 (d) –2 – x = 6
2 2
4. Solve the following equations:

3y x x 1
(a) = 12 (b) – 5 = 1 (c) + 2 = 11 (d) 2x – = 1
4 5 7 4

Transposing a Term of an Equation

In an equation a term can be shifted from one side to the other side by changing its sign. This
process is known as transposition. A positive term changes to negative and a negative term changes
to positive on transposition. Transposing a number is equivalent to adding (or subtracting) the

same number to both sides. Hence it does not affect the equation in any way.
Example 11: Solve the following equations:
7 43 q 5k 5 2b
(a) 3y + = (b) + 7 = 7 (c) = (d) – 5 = 3
3 3 4 3 3 3

7 43 q
Solution: (a) 3y + = (b) + 7 = 7
3 3 4
43 7 q
⇒ 3y = – ⇒ = 7 – 7
3 3 4
43 – 7 q
⇒ 3y = 3 ⇒ = 0
4
36 ⇒ q = 0
⇒ 3y =
3
⇒ 3y = 12 2b
⇒ y = 4 (d) 3 – 5 = 3

5k 5 ⇒ 2b = 3 + 5
(c) = 3
3 3

5 ⇒ 2b = 8
⇒ 5k = × 3 3
3
⇒ 5k = 5 ⇒ b = 8 × 3
5 2
⇒ k =
5 ⇒ b = 12
⇒ k = 1

109