Page 115 - Start Up Mathematics_7

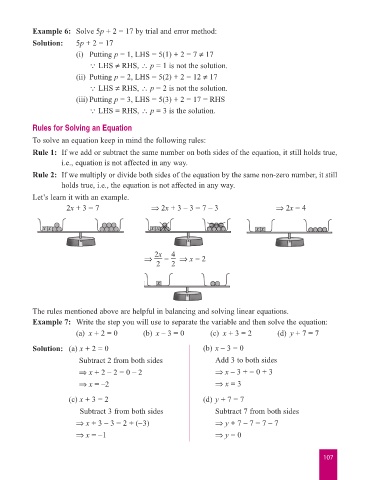
Example 6: Solve 5p + 2 = 17 by trial and error method:

Solution: 5p + 2 = 17
(i) Putting p = 1, LHS = 5(1) + 2 = 7 ≠ 17
 LHS ≠ RHS, ∴ p = 1 is not the solution.

(ii) Putting p = 2, LHS = 5(2) + 2 = 12 ≠ 17
 LHS ≠ RHS, ∴ p = 2 is not the solution.
(iii) Putting p = 3, LHS = 5(3) + 2 = 17 = RHS

 LHS = RHS, ∴ p = 3 is the solution.

Rules for Solving an Equation

To solve an equation keep in mind the following rules:
Rule 1: If we add or subtract the same number on both sides of the equation, it still holds true,
i.e., equation is not affected in any way.

Rule 2: If we multiply or divide both sides of the equation by the same non-zero number, it still
holds true, i.e., the equation is not affected in any way.

Let’s learn it with an example.
2x + 3 = 7 ⇒ 2x + 3 – 3 = 7 – 3 ⇒ 2x = 4

x x x x x x

2x 4
⇒ = ⇒ x = 2
2 2

x

The rules mentioned above are helpful in balancing and solving linear equations.
Example 7: Write the step you will use to separate the variable and then solve the equation:

(a) x + 2 = 0 (b) x – 3 = 0 (c) x + 3 = 2 (d) y + 7 = 7

Solution: (a) x + 2 = 0 (b) x – 3 = 0
Subtract 2 from both sides Add 3 to both sides

⇒ x + 2 – 2 = 0 – 2 ⇒ x – 3 + = 0 + 3
⇒ x = –2 ⇒ x = 3

(c) x + 3 = 2 (d) y + 7 = 7

Subtract 3 from both sides Subtract 7 from both sides
⇒ x + 3 – 3 = 2 + (–3) ⇒ y + 7 – 7 = 7 – 7

⇒ x = –1 ⇒ y = 0

107