Page 82 - Start Up Mathematics_6

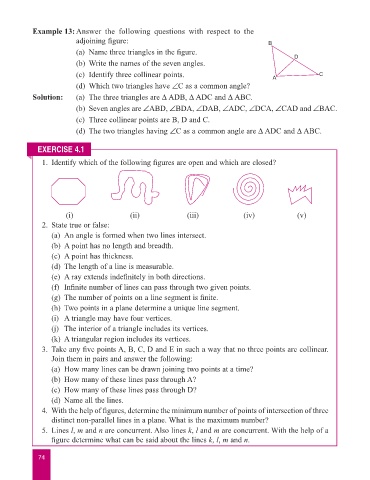
Example 13: Answer the following questions with respect to the
adjoining figure: B

(a) Name three triangles in the figure.
D
(b) Write the names of the seven angles.
(c) Identify three collinear points. A C

(d) Which two triangles have ∠C as a common angle?
Solution: (a) The three triangles are ∆ ADB, ∆ ADC and ∆ ABC.
(b) Seven angles are ∠ABD, ∠BDA, ∠DAB, ∠ADC, ∠DCA, ∠CAD and ∠BAC.

(c) Three collinear points are B, D and C.
(d) The two triangles having ∠C as a common angle are ∆ ADC and ∆ ABC.

EXERCISE 4.1
1. Identify which of the following figures are open and which are closed?

(i) (ii) (iii) (iv) (v)
2. State true or false:
(a) An angle is formed when two lines intersect.

(b) A point has no length and breadth.
(c) A point has thickness.
(d) The length of a line is measurable.
(e) A ray extends indefinitely in both directions.
(f) Infinite number of lines can pass through two given points.
(g) The number of points on a line segment is finite.
(h) Two points in a plane determine a unique line segment.
(i) A triangle may have four vertices.

(j) The interior of a triangle includes its vertices.
(k) A triangular region includes its vertices.
3. Take any five points A, B, C, D and E in such a way that no three points are collinear.
Join them in pairs and answer the following:
(a) How many lines can be drawn joining two points at a time?
(b) How many of these lines pass through A?
(c) How many of these lines pass through D?
(d) Name all the lines.

4. With the help of figures, determine the minimum number of points of intersection of three
distinct non-parallel lines in a plane. What is the maximum number?
5. Lines l, m and n are concurrent. Also lines k, l and m are concurrent. With the help of a
figure determine what can be said about the lines k, l, m and n.

74