Page 80 - Start Up Mathematics_6

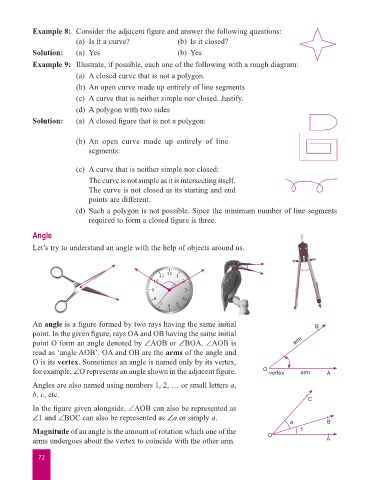
Example 8: Consider the adjacent figure and answer the following questions:
(a) Is it a curve? (b) Is it closed?

Solution: (a) Yes (b) Yes
Example 9: Illustrate, if possible, each one of the following with a rough diagram:
(a) A closed curve that is not a polygon.

(b) An open curve made up entirely of line segments
(c) A curve that is neither simple nor closed. Justify.

(d) A polygon with two sides
Solution: (a) A closed figure that is not a polygon:

(b) An open curve made up entirely of line
segments:

(c) A curve that is neither simple nor closed:
The curve is not simple as it is intersecting itself.
The curve is not closed as its starting and end
points are different.
(d) Such a polygon is not possible. Since the minimum number of line segments
required to form a closed figure is three.

Angle
Let’s try to understand an angle with the help of objects around us.

An angle is a figure formed by two rays having the same initial B
point. In the given figure, rays OA and OB having the same initial
point O form an angle denoted by ∠AOB or ∠BOA. ∠AOB is arm
read as ‘angle AOB’. OA and OB are the arms of the angle and
O is its vertex. Sometimes an angle is named only by its vertex,
for example, ∠O represents an angle shown in the adjacent figure. O vertex arm A
Angles are also named using numbers 1, 2, … or small letters a,
b, c, etc.
C
In the figure given alongside, ∠AOB can also be represented as
∠1 and ∠BOC can also be represented as ∠a or simply a.
a B
Magnitude of an angle is the amount of rotation which one of the O 1
arms undergoes about the vertex to coincide with the other arm. A

72