Page 67 - Start Up Mathematics_6

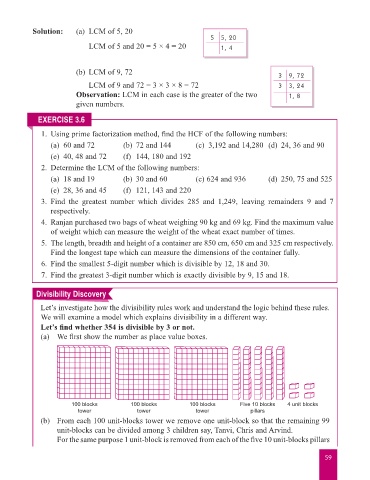
Solution: (a) LCM of 5, 20
5 5, 20
LCM of 5 and 20 = 5 × 4 = 20 1, 4

(b) LCM of 9, 72 3 9, 72
LCM of 9 and 72 = 3 × 3 × 8 = 72 3 3, 24
Observation: LCM in each case is the greater of the two 1, 8
given numbers.

EXERCISE 3.6
1. Using prime factorization method, find the HCF of the following numbers:

(a) 60 and 72 (b) 72 and 144 (c) 3,192 and 14,280 (d) 24, 36 and 90
(e) 40, 48 and 72 (f) 144, 180 and 192

2. Determine the LCM of the following numbers:
(a) 18 and 19 (b) 30 and 60 (c) 624 and 936 (d) 250, 75 and 525
(e) 28, 36 and 45 (f) 121, 143 and 220
3. Find the greatest number which divides 285 and 1,249, leaving remainders 9 and 7
respectively.

4. Ranjan purchased two bags of wheat weighing 90 kg and 69 kg. Find the maximum value
of weight which can measure the weight of the wheat exact number of times.
5. The length, breadth and height of a container are 850 cm, 650 cm and 325 cm respectively.
Find the longest tape which can measure the dimensions of the container fully.
6. Find the smallest 5-digit number which is divisible by 12, 18 and 30.

7. Find the greatest 3-digit number which is exactly divisible by 9, 15 and 18.

Divisibility Discovery
Let’s investigate how the divisibility rules work and understand the logic behind these rules.
We will examine a model which explains divisibility in a different way.
Let’s find whether 354 is divisible by 3 or not.
(a) We first show the number as place value boxes.

100 blocks 100 blocks 100 blocks Five 10 blocks 4 unit blocks
tower tower tower pillars
(b) From each 100 unit-blocks tower we remove one unit-block so that the remaining 99
unit-blocks can be divided among 3 children say, Tanvi, Chris and Arvind.
For the same purpose 1 unit-block is removed from each of the five 10 unit-blocks pillars

59